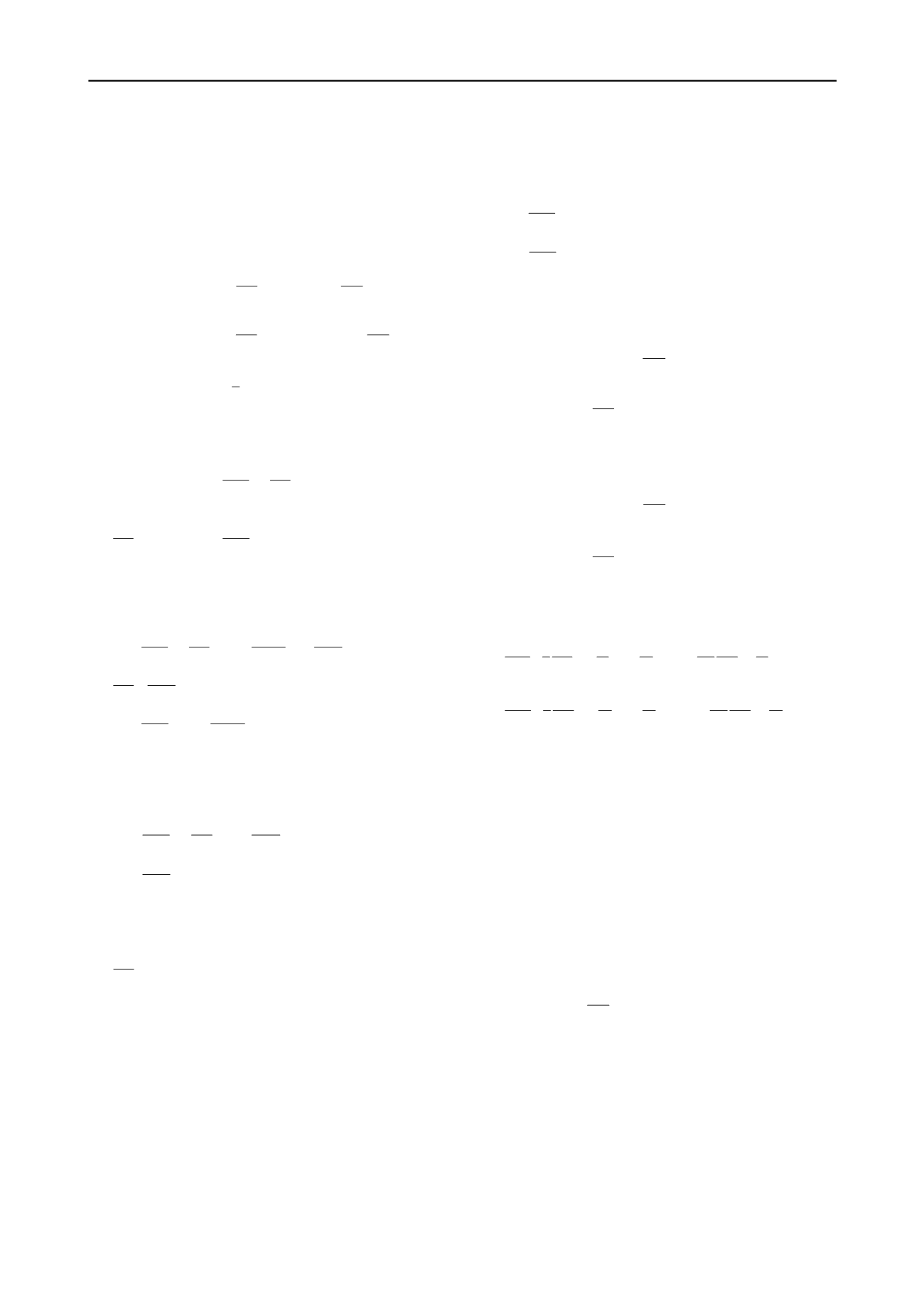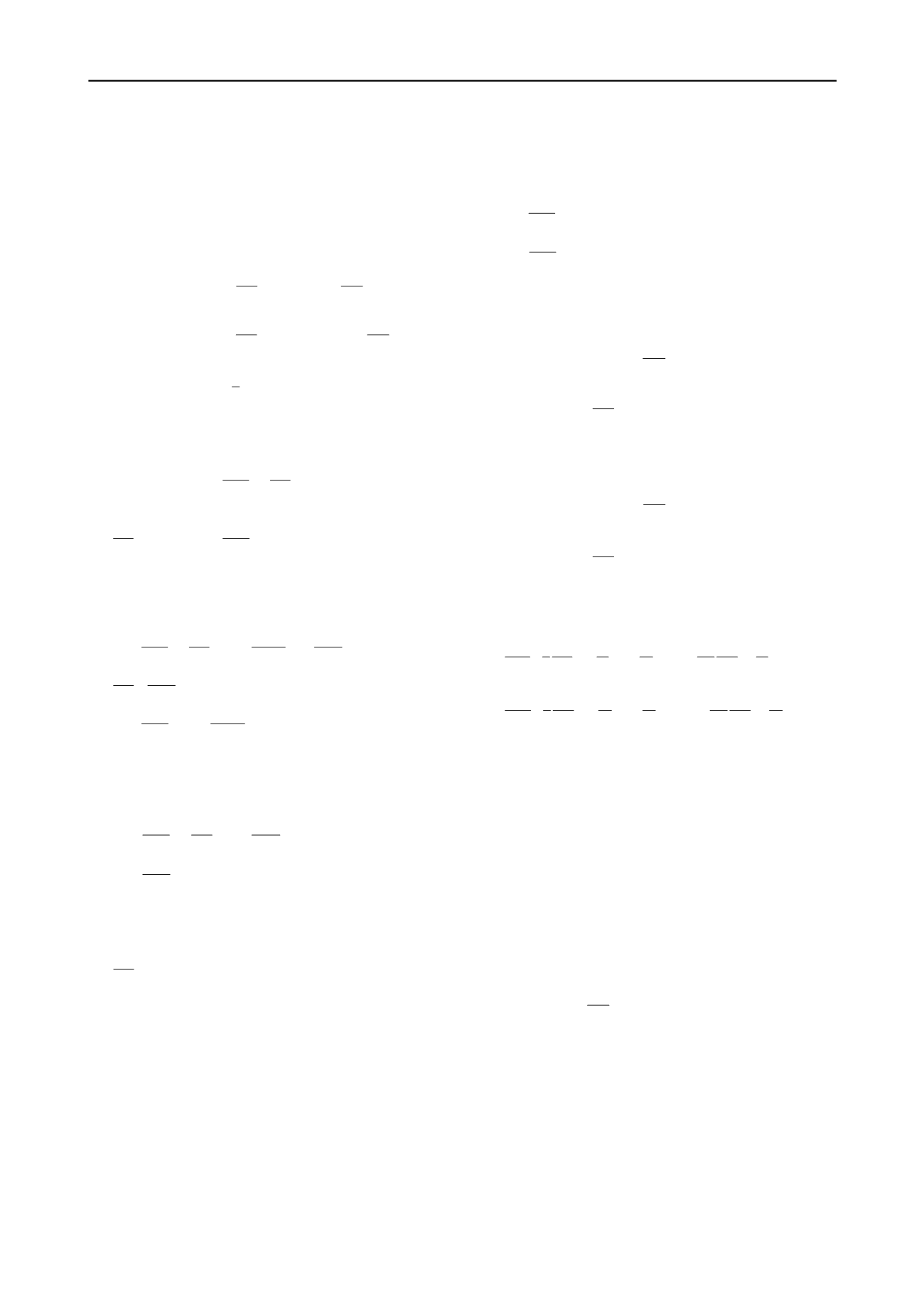
2857
Technical Committee 212 /
Comité technique 212
2
2
,
2
2
2
,
0
p
p
s i
r
r
i
s i
r
p
p
r
G
rdr
t
G
rdr r
z
p
z L
L
(8)
2
2
,
,
,
,
,
2
,
,
(
2 )
+2 (
)
2 (
)
1
+(
3 ) (
)
p
p
p
p
p
r
i
s i
s i
s i
r
r
r
s i
r
s i
r
r
r
s i
s i
r
r
d
d
k
G r
dr G r
dr
dr
d
d dr G
dr
dr
dr
G
dr
r
dr
(9)
The boundary conditions for the differential equations are
given below:
At
z =
0:
3
1
1
1
0
1
3
2
1
1
0
2
or
and
or
p p
p p
a
d w dw
w w
E I
t
F
dz
dz
dw
d w E I
M
dz
dz
a
(10)
At
z = H
i
< L
p
:
1
3
3
1
1
3
3
1
2
2
1
2
2
i
i
i
i
i
p p
i
p p
i
i
i
i
i
p p
p p
w w
d w dw
d w dw
E I
t
E I
t
dz
dz
dz
dz
dw dw
dz dz
d w
d w
E I
E I
dz
dz
1
i
(11)
At
z = L
p
, for free-base pile base:
1
3
1
1
3
2
2
0
i
i
i
i
p p
i
i
i
p p
w w
d w dw dw
E I
t
t
dz
dz
dz
d w E I
dz
i
(12)
At
z = L
p
, for fixed-base pile base:
1
0
0
i
i
i
w w
dw
dz
(13)
As
z
՜
∞
,
0
i
w
(14)
The above differential equations are solved analytically after
applying the boundary conditions to obtain the response of
piles. The details of the solution can be found in Basu and
Salgado (2008) and Basu et al. (2009).
3.4
Soil displacement functions
The differential equations for the soil displacement functions
in the case of rectangular piles are given by:
2
2
2
2
0
0
x
x
x x
y
y
y y
dp
q
dx
d
p
q
dy
(15)
where,
1
1
2
,
,
1
(
2 )
i
i
H
n
x
s i
s i
i
y
i
H
2
p
G w dz
dy
(16)
1
1
2
1
2
,
1
2
1
2
,
1
i
i
i
i
H
n
y
x
s i
i
i
H
H
n
i
s i
y
i
H
d
q
G w dz
dy
dy
dw G
dz
dy
dz
2
(17)
1
1
2
,
,
1
(
2 )
i
i
H
n
y
s i
s i
i
x
i
H
p
G w dz
dx
(18)
1
1
2
1
2
,
1
2
1
2
,
1
i
i
i
i
H
n
x
y
s i
i
i
H
H
n
i
s i
x
i
H
d
q
G w dz
dx
dw G
dz
dz
dx
dx
(19)
The differential equations for the soil displacement functions
in the case of circular piles are given by:
2
2
2
2
2
3
1
2
1
2
2
2
2
2
2
5
6
4
4
2
1
1
r
r
r
p
r
p
d
d
d
dr r dr
r
r
r dr
r
d
d
d
dr r dr
r
r
r dr
r
(20)
where
2
2
2
1
4
1 2
1 3
2
3
/
, ( / )
/
,
(
) /
1
s
s
p
s
s
s
s
m m r
n m
m m m
2
2
2
4
4
2 5
2 6
2
3
/
, ( / )
/
,
(
) /
s
2
and
s
s
p
s
s
s
s
m m r
n m
m m m
s
w dz
dz
w dz
w dz
with
1
1
2
1
,
,
1
(
2 )
i
i
H
n
s
s i
s i
i
i
H
m
G
H
(21)
1
1
2
2
,
1
i
i
n
s
s i
i
i
H
m G w
H
(22)
1
1
2
3
,
1
i
i
n
s
s i
i
i
H
m
(23)
1
1
2
4
,
,
1
(
3 )
i
i
H
n
s
s i
s i
i
i
H
m
G
(24)
1
2
1
,
1
i
i
H
n
i
s
s i
i
H
dw
n G
dz
dz
(25)
As mentioned earlier, these displacement functions are equal
to unity at the pile-soil interface and they are equal to zero at the
boundaries of the domain at infinity.
The above differential equations of the soil displacement
functions for rectangular piles can be solved analytically as
shown in Basu and Salgado (2008), while the coupled
differential equations that govern the soil displacement
surrounding the circular piles can be solved numerically using
the finite difference method as shown in Basu et al. (2009).
As evident from Eqs. 5, 15, and 20, the responses of the pile
and soil to the lateral loading are interrelated. Therefore, these
differential equations are solved simultaneously following an