2966
Proceedings of the 18
th
International Conference on Soil Mechanics and Geotechnical Engineering, Paris 2013
Proceedings of the 18
th
International Conference on Soil Mechanics and Geotechnical Engineering, Paris 2013
compression modulus and
is the unit weight of water),
is
the consolidation time,
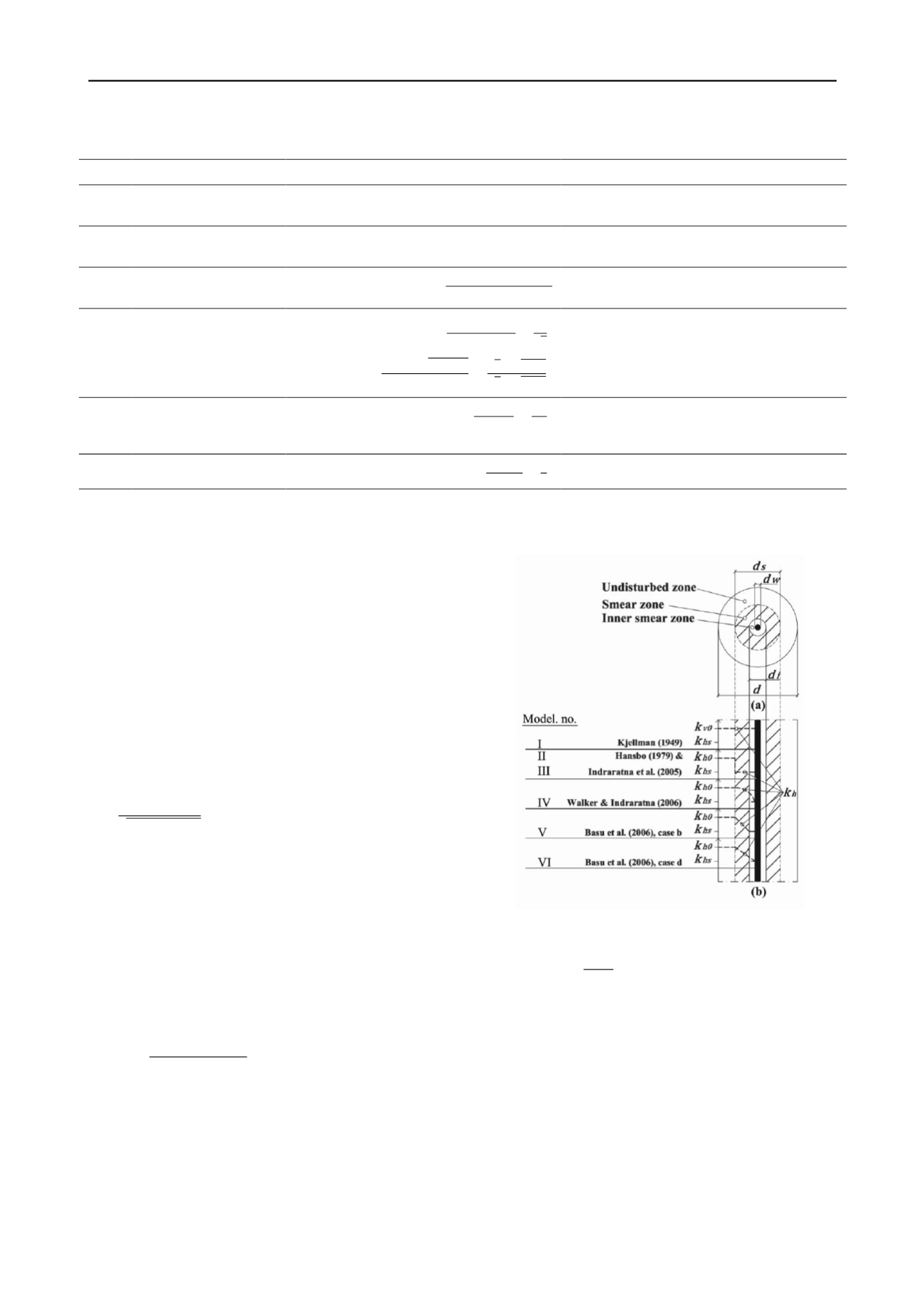
is the diameter of the assumed unit
cell dewatered by a single drain (cf. Figure 1) and the
expression
is dependent on the model.
1 METHODS
The characteristics and formulations of the expression
in the
six investigated models are presented in Table 1 and Figure 1b.
Denoting the variables in Eq. 1 and in the formulations of
(i.e.
⁄
⁄
) as
, the partial
derivative of
with respect to the variable
, i.e.
⁄
, can
be obtained and the influence of each variable on
can be
assessed:
⁄
√∑ (
⁄ )
(2)
This was done for all of the aforementioned models, assigning
( )
metres and for values of
resulting in
assessments of
ranging from 0 to 1. In addition, the
uncertainties in the assessments of
(expressed as the variance,
) were evaluated. In these analyses, the variables
,
,
and
⁄
were treated stochastically, while the other variables
were assumed to be deterministic, and the variances in the four
variables were propagated through Eq. 1 via second order
Taylor series approximations (e.g. Fenton and Griffiths, 2008
pp. 30-31). The contribution to
from each variable was
then assessed as (e.g. Christian et al. 1994):
(
⁄ )
∑ [(
⁄ )
]
(3)
Values assigned to the variables adopted in the analyses are
presented in Table 2.
2 RESULTS
2.1
Assessments of U from the six models
In Figure 2, the degrees of consolidation
assessed from the
six models are presented as a function of
for the three values
of
. In the figure, a span representing two standard deviations
(SD), i.e.
√
, is presented for
1.1 m. The
appearance is similar for the other two values of
. The curves
plot at a close distance and well within the span of 2xSD for the
respective values of
, i.e the uncertainties in the variables had
a greater impact on the assessed value of
than the choice of
model.
Table 1. Characteristics and formulations of
F
in the investigated models (valid for
10
A
and neglecting well resistance)
no.
Characteristics
Formulation
A
Reference and comments
I
No smear zone,
is
used instead of
B
( )
Kjellman (1949), smear effects accounted for by
adopting
instead of
II
and constant
in the smear zone
( ⁄ ) ( )
Hansbo (1979), equal to model no. I for
1
III
Equal to no. II,
dependent on the void ratio
(
⁄ )
⁄
Indraratna et al. (2005), valid for normally con-
solidated clays, equal to model no. II for
⁄
IV
Parabolic variation of
in the smear zone
( ⁄ ) ( )
(
) ( √ )
( )√ ( )
(
) ( √ √
√ √ )
Walker and Indraratna (2006)
V
in the inner
smear zone thereafter
linear variation
( ⁄ ) ( ) ⁄ ( )
Basu et al. (2006), case b, equal to model no. VI
for
1
VI
Linear variation
( ⁄ ) ⁄ ( )
Basu et al. (2006), case d
A
⁄
;
&
=initial stress & stress from the applied load;
&
=compression & permeability indices;
⁄
B
1.5 was used based on suggestions in Tavenas et al. (1983) for the anisotropy in permeability in homogeneous clays.
Figure 1. a) Plan view of the unit cell; b) Vertical section of the unit cell
and illustration of the analytical models investigated.


