3348
Proceedings of the 18
th
International Conference on Soil Mechanics and Geotechnical Engineering, Paris 2013
Figure 1. Schematic response of a pile subjected to heating and cooling,
aft
nterface is also of
int
ture dependent in void ratio–mean effective stress
sp
positive
va
res OC clay
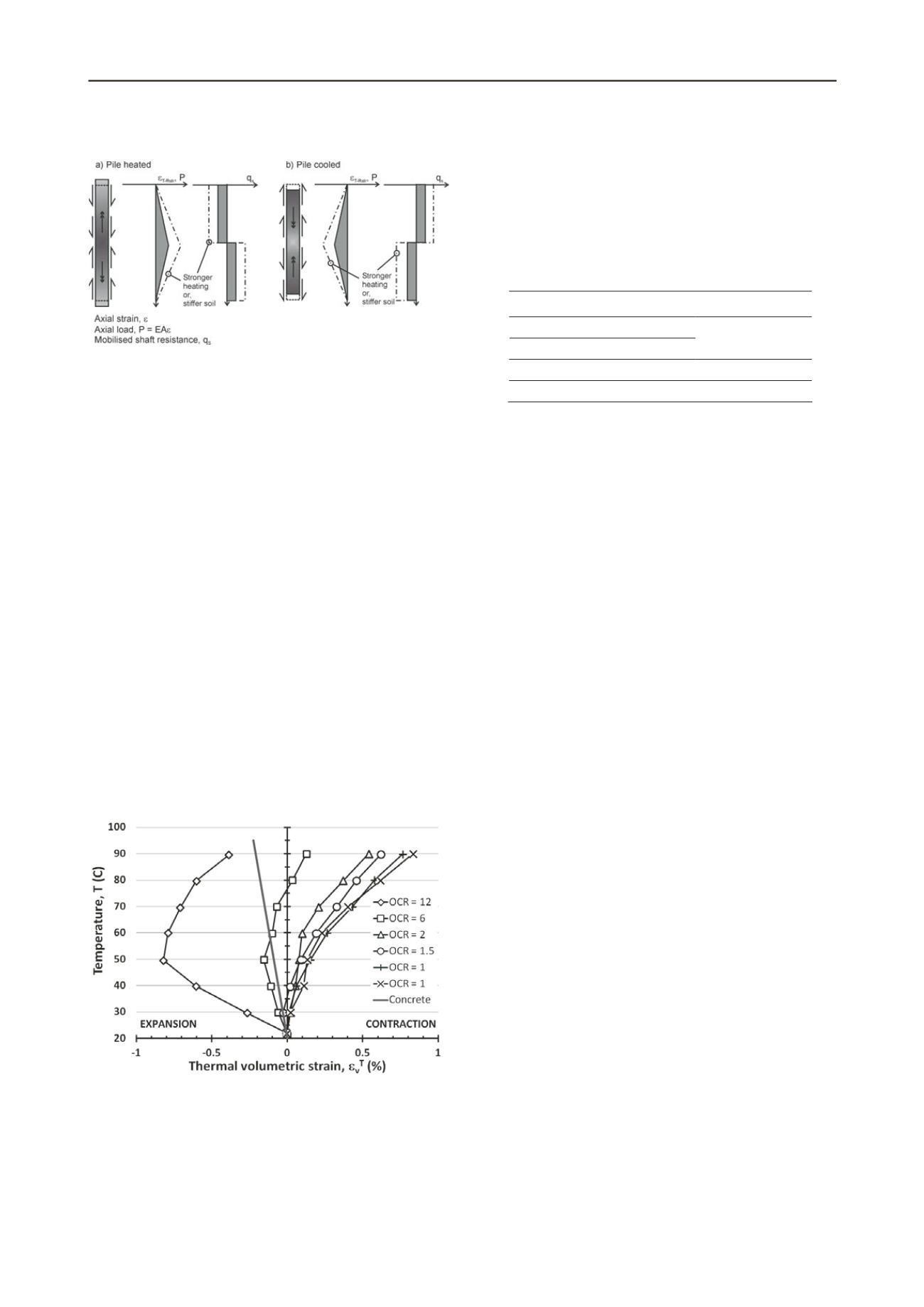
becomes contractive, e.g. at about 50°C in Fig. 2.
hermal volumetric response of Kaolin, after
Ce
xpand more
tha
ent on the type of aggregates that
are used in the concrete mix.
f thermal expansion,
quoted for
ey soils, concrete and water.
(E-5, °K
-1
)
er Bourne-Webb et al. 2013.
In addition to the general impact on soil behaviour, the
impact of temperature cycles at the pile-soil i
erest in the case of energy geo-structures.
Experimentally, the effect of temperature on the mechanical
behaviour of clayey soils has been found to be equivalent to that
of strain rate, Marques et al., 2004. The effects are permanent
and the soil behaviour can be described by a unique stress-
strain-temperature law. In addition, it is found that while peak
undrained strength increases with decreasing temperature, the
critical state failure envelope is unique in stress space but
tempera
ace.
The thermal volumetric response of clay soil has been
examined in a number of laboratory investigations (Campanella
& Mitchell 1967; Baldi et al. 1988; Cekerevac & Laloui 2004)
and it was found that the volume change of a clay sample in
response to a change in temperature depends on the over-
consolidation ratio (OCR). When heated, normally consolidated
soil (OCR = 1) contracts (implying a negative coefficient of
thermal expansion) and as the OCR increases, the soil becomes
increasingly less contractive with moderately to highly over-
consolidated (OC) clay being expansive, i.e. with
lues of the coefficient of thermal expansion, Fig. 2.
The testing also suggests that the thermal expansion of OC
clay is reversible but there is a limit to the range of temperatures
over which this occurs. At higher temperatu
Figure 2. Effect of OCR on t
kerevac & Laloui, 2004.
Thus, a heavily OC clay such as the London Clay that
supported the Lambeth College test pile, should e
n concrete, perhaps by a factor of two or more.
The relative deformation of the pile with respect to the soil,
in response to temperature change, is thought to be the source of
the observed changes in pile response. Therefore, in this study,
the effect of variations in the soil coefficient of thermal
expansion relative to that of a concrete pile undergoing heating
was evaluated. Table 1 summarises some typical values of this
parameter for stiff over-consolidated clay found in the literature,
and which formed the basis for selecting values for the
numerical analyses, Table 2. Also, detailed in Table 1 are values
of the coefficient of thermal expansion for water and concrete.
The latter is primarily depend
able 1. Typical values of volumetric coefficient o
T
two clay
Material
Boom clay
Opalinus clay
4 to 6
Concrete
2 to 4
Water (at 22°C)
27
2 BASIS FOR FINITE ELEMENT ANALYSES
e of 60 m (2L) and 90 m (3L) respectively, Cruz Silva,
20
se on the pile-
so
vertical movement is
pr
ground surface, although the latter is probably closest to reality.
respect to soil response which can be
str
s), more realistic thermal loading and boundary
co
2.1
Geometry and boundary conditions
A single pile with a diameter, D of 1.0 m and length, L of 30 m
has been modelled in the program ADINA V8.5.0 assuming
axisymmetry. After initial verification analyses, the side and
bottom boundaries of the finite element mesh were set at a
distanc
12.
No interface elements were introduced between the pile and
soil solid elements, implying that the contact was perfectly
rough. This also implies that the stiffness in the interface zone is
the same as that for the soil, whereas the respon
il interface is known to be significantly stiffer.
Mechanical loading of the pile was modelled by applying a
boundary pressure (6 MPa) that resulted in a pile settlement of
about 1% of the pile diameter, i.e. about 10 mm. Displacement
boundary conditions fix horizontal movement on the bottom
and the two side boundaries while
evented only on the bottom boundary.
Thermal loading of the pile was modelled by the application
of an increment of temperature
T=+30°C to all the elements
making up the pile under steady state heat flow conditions. It is
acknowledged that this is a simplification with respect to the
actual temperature distribution in the pile cross-section and
surrounding soil with time, but is considered to be reasonable
with respect to the temperature along the pile which has been
found to be almost constant, Bourne-Webb et al. 2009. Thermal
boundary conditions ensured zero heat flow on the model
centreline, and zero temperature change on the side and lower
boundaries. Two scenarios were examined regarding the
thermal boundary condition along the ground surface: zero heat
flow and constant temperature. The resultant temperature fields
are shown in Fig. 3. Again, these are acknowledged to be
significant simplifications of the actual thermal conditions at the
2.2
Material parameters
In these analyses, both the pile and the soil were assumed to be
elastic. This is considered to be a reasonable assumption for the
structural element but is acknowledged to be a great
simplification with
ongly nonlinear.
However, the aim of this study was to examine the pile
response to temperature change on a simplified basis; additional
layers of complexity may be added subsequently, including e.g.
an interface with finite shear resistance, nonlinear TM/THM
soil model(
nditions.


