3349
Technical Committee 307 + 212 /
Comité technique 307 + 212
The adopted model parameters are shown in Table 2. In all
the analyses undertaken,
for the concrete was held constant
with a value of 3.0E-5°K
-1
(note that the coefficient of linear
thermal expansion,
=
/3). The values of
assumed for the
so
order to
d thermal
e thermal
Table 2.
med for num
ysis.
Concrete
Soil
il were zero, half and double that for the concrete;
representing a moderately OC clay.
In addition, the Young’s modulus of the soil was increased
by a factor of two from the base value of 30 MPa, in
assess the effect of this parameter on the predicte
response of the pile.
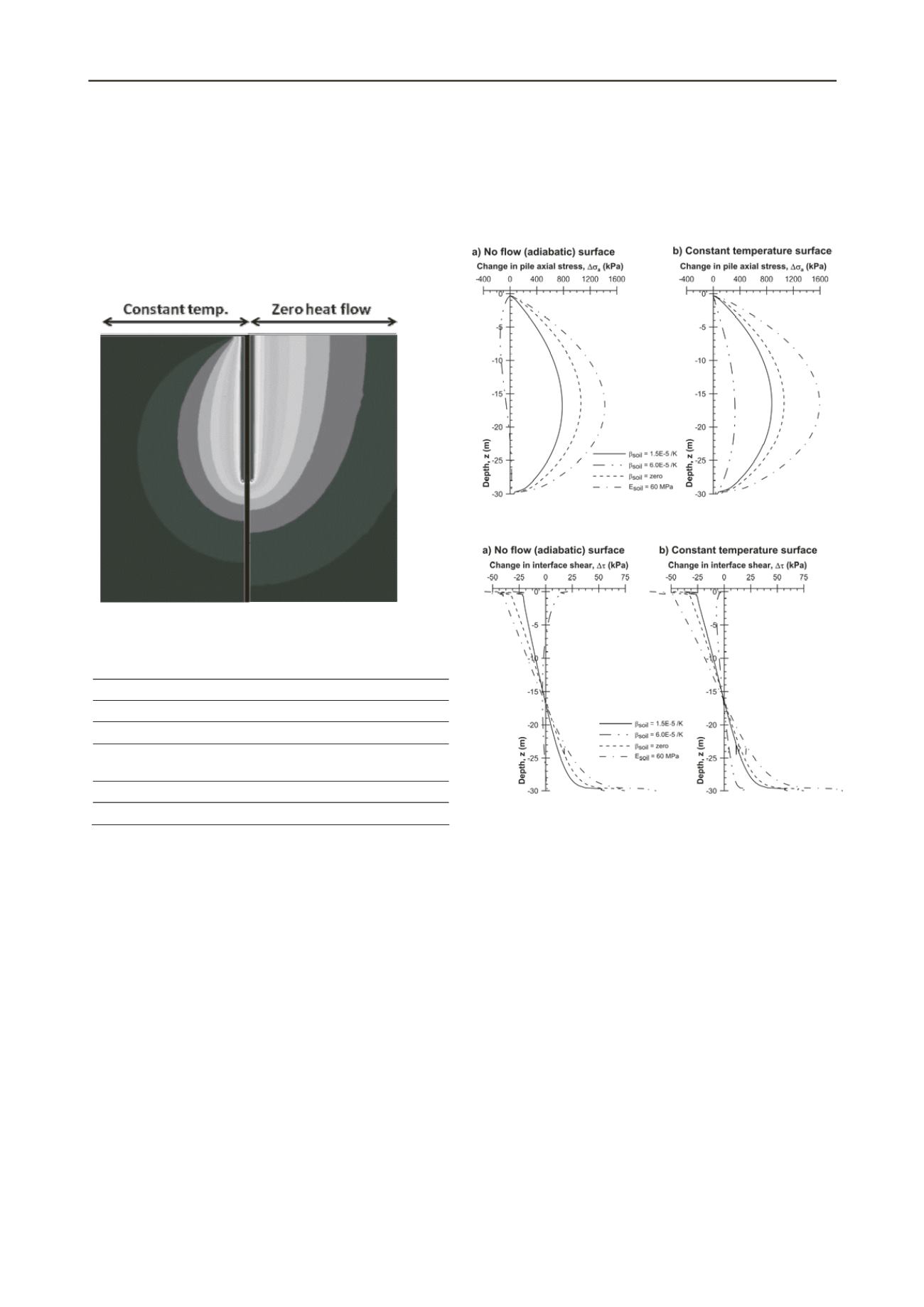
Figure 3. Steady-state temperature field as function of surfac
oundary condition (contour interval: 2°C)
b
Material parameters assu
erical anal
Parameter
Young’s modulus, E (MPa)
30000
30 or 60
Poisson’s ratio,
( - )
0.3
0.3
Coefficient of volumetric thermal
-1
expansion,
(E-5, °
K
)
3.0
0,
r
1.5 o
6.0
Thermal conductivity, k (kJ/hr.m.K)
8.4
4.0
Volumetric heat capacity,
c
p
(kJ/m
3
.K)
1950
1500
3 PREDICTIONS
3.1
Coefficient of thermal expansion
The effect of changes in the value of the coefficient of
volumetric thermal expansion,
of the soil, the stiffness of the
soil and the thermal boundary condition on the ground surface
of the model are illustrated, in terms of changes in pile axial
stress, Fig. 4 and pile-soil interface shear, Fig. 5.
When comparing the plots, the dashed line for the
= zero
case (the soil is thermally inert) provides a baseline for
comparison, as the results are independent of the thermal
boundary condition on the ground surface.
When the soil is less thermally expansive than the pile, i.e.
= 1.5E-5°K
-1
and zero, heating the pile led to compressive axial
stress with the maximum stress change for each
-value
equating to about +12% and +15% of the stress that would be
mobilised if the pile was fully restrained, P
fix
(Table 3). The
constant temperature boundary condition results in slightly
greater (1 to 2%) restraint of the pile thermal expansion and
thus, higher compressive axial stress are developed.
The effect of the thermal boundary condition on the ground
surface becomes clearer when the soil is assumed to be more
expansive than the pile (
= 6.0E-5°K
-1
); when a zero heat flow
condition was assumed, the pile went into tension (max. stress
about -2% of P
fix
) however, as identified above, the use of a
constant temperature boundary condition resulted in greater
restraint and the resulting stress changes were compressive
(m . stress about +5% of P
fix
) along the entire length of the
and therefore the variation in
r with depth).
e coefficient of volumetric thermal
e of the model being elastic and the
int
iles inferred from observations in test
pil
ax
pile.
Figure 4. Change in pile axial stress due to temperature change of
+30°C, Cruz Silva 2012.
Figure 5. Change in pile-soil interface shear stress due to temperature
change of +30°C, Cruz Silva 2012.
The shape of the profiles of predicted axial stress change
(approx. parabolic) in Fig. 4 are directly related to the shape of
the profile of mobilised friction at the pile-soil interface, Fig. 5
which is approximately linear (note that in Fig. 1 the mobilised
friction was assumed constant
axial stress was linea
Here again the effect of th
expansion of the soil and the thermal boundary condition on the
ground surface is seen. As the contrast in
-values of the pile
and the soil increases, the magnitude of the predicted change in
shear stress on the pile-soil interface, and the constant
temperature condition leads to larger changes in shear stress
compared to the zero heat flow condition.
As a consequenc
erface not being modelled explicitly, i.e. with an appropriate
stiffness and limiting strength, the shape of the interface friction
(shear stress) profiles differs from that expected based on the
simple model in Fig. 1 (which effectively assumes perfect
plasticity) and the prof
es, Amatya et al. 2012. The variation in shear stress along the
pile-soil interface suggested here is only likely to be correct
while the maximum stress values are below the yield strength
on the interface.


