2696
Proceedings of the 18
th
International Conference on Soil Mechanics and Geotechnical Engineering, Paris 2013
4 0
c
ti
p p
E
IE
L
dz zE
D
E
e
usually derived from bending moment profiles measured by
strain gauges along the pile. However, a few full-scale tests on
instrumented piles were reported in the literature with
successful derivation of the P-Y curves from double
differentiation and integration of the bending moment profile.
The main difficulty in deriving these curves is due to the high
sensitivity of the lateral soil reaction P to the experimental
conditions as well as to the method of fitting and differentiation
of the bending moments (Bouafia and Garnier, 1991).
The method of construction of the P-Y curves presented in
this paper in based on the interpretation of several full-scale
lateral loading tests on fully instrumented piles carried out in a
variety of quite homogeneous soils in France. A detailed
description of the experimental sites, the test piles and the
interpretation of the experimental P-Y curves was given by
Bouafia (2005) and Bouafia and Lachenani (2005). The paper
rather focuses on the formulation of K
E
and K
p
, the validation of
the proposed P-Y curves by predicting the behaviour of test
piles reported in the literature and the discussion of the concept
of the critical pile deflection.
D
ti
e
c
ti
0
)(
1
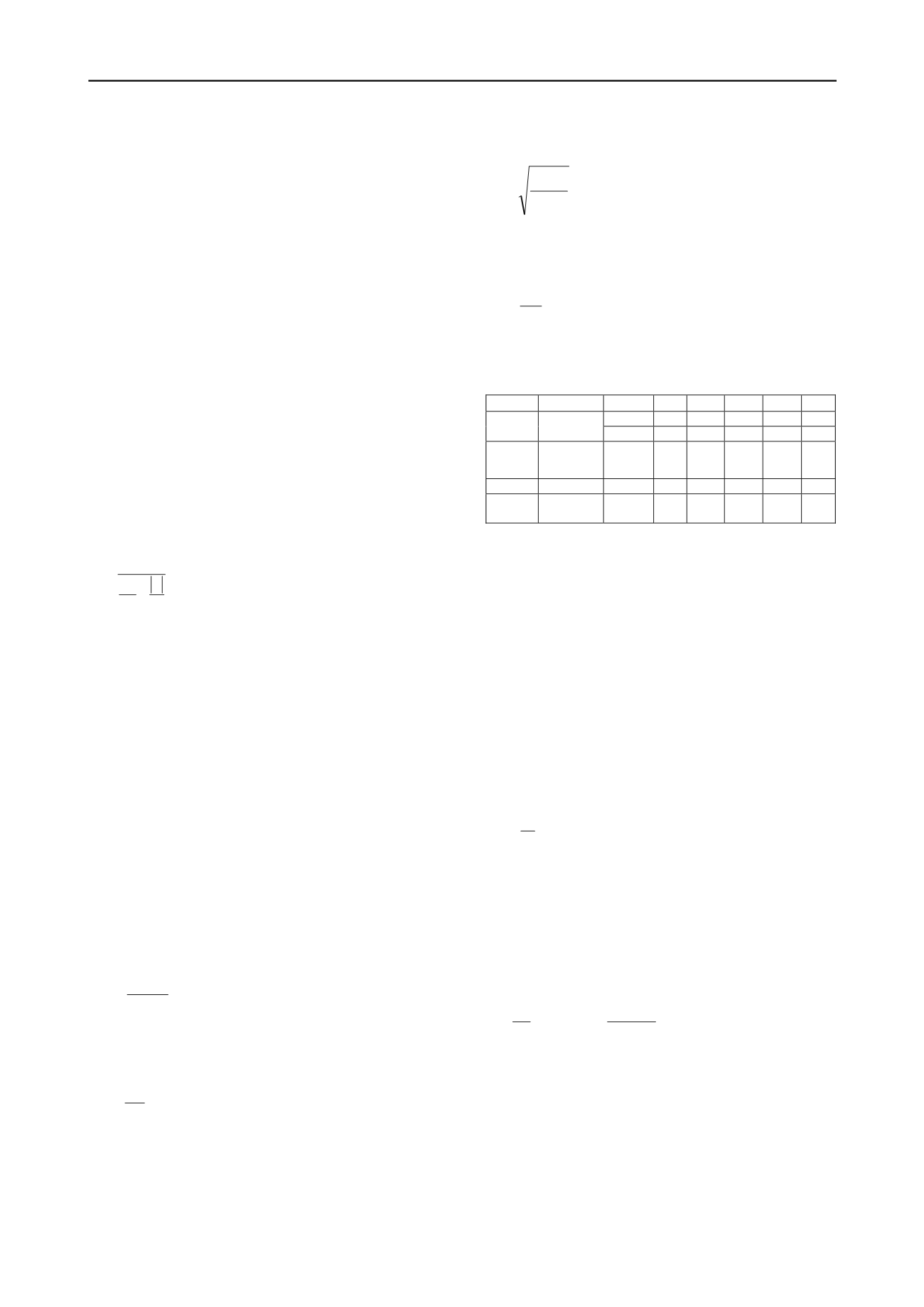
2 FORMULATION OF THE P-Y CURVE
The P-Y curve at a given depth is described by a usual
hyperbolic formulation as follows (Reese, 1971; Garassino,
1976; Georgiadis et al, 1992):
(5)
The parameters E
ti
and P
u
may be computed according to
equations (3) and (4) respectively.
The modulus number and the lateral resistance factor were
found varying as a power with the pile/soil stiffness ratio K
R
,
such as (Bouafia, 2007):
K
E
= aK
R
n
(6)
K
p
= b + cK
R
m
(7)
Table 1 summarises the values of coefficients a, b, c, n and
m. Due to the limited data regarding the behaviour of
experimental piles in organic clays and in silty soils it was not
possible to analyse K
E
and K
p
for such soils. Average values
were nevertheless proposed in Table 1.
It should be emphasized that the influence of the lateral
pile/soil stiffness on the P-Y curve was not accounted for by the
current methods of construction of P-Y curves which simply
correlate the parameters of the P-Y curve to those of the PMT
test (Gambin, 1979).
The lateral pile/stiffness may be defined as follows:
4
.
.
e c
p p
R
DE
IE
K
(8)
E
c
is the characteristic PMT modulus defined as the average
value of the PMT moduli such as:
e
D
M
e
c
dz E
D
E
0
.
1
(9)
D is the embedded length of the pile and D
e
is the effective
embedded length of the pile, beyond which the pile segments do
not deflect. It is computed as:
D
e
= min{D, πL
0
} (10)
The elastic length (or the transfer length) L
0
is given by:
(11)
E
ti
c
is the characteristic lateral reaction modulus of the
equivalent homogeneous soil (or the average lateral reaction
modulus) given by:
(12)
Table 1. Values of coefficients a, b, c, n and m
Soil
D/B
K
R
a
n
b
c
m
≥ 0.01 0.33 - 0.5
0.0
3.0
0.5
Sand
D/B ≥ 10
< 0.01 3.40 0.0
0.0
0.31 0.0
Clay
D/B ≥ 5
1.85
- 0.2
0.3
1.0
1.0
Silt
5.50 0.0
2.30
0.0
0.0
Organic
clay
3.70 0.0
0.14
0.0
0.0
3 METHODOLOGY OF CONSTRUCTION OF THE P-Y
u
ti
P
Y
E
y
P
1
CURVE
The following step-by-step procedure helps using the method to
define the P-Y curves parameters:
1. Subdivide the soil along the pile into N horizontal slices
enough thin so that the PMT data (E
m
, P
l
) may be considered
varying linearly within any slice. The value of any of these
parameters at the mid-slice is then considered as representative
within the slice.
2. If the soil is composed of n layers around the pile, compute
the average PMT modulus E
M
i
for each layer i supposed thick of
h
i
as follows:
i
h
M
i
i
M
dz E
h
E
0
.
1
(13)
3. Assume D
e
=D that is to say the pile is assumed to be semi-
rigid or rigid.
4. Compute the characteristic soil modulus E
c
by equation 9.
For practical purposes, replace the integration formula by that of
the summation of trapezes:
e
ni
i
i
i
M
D
M
e
c
D
hE
dz z E
D
E
e
1
0
)(
1
(14)
5. Compute the lateral pile/soil stiffness ratio K
R
according to
the equation (8).
6. Compute the modulus number K
E
i
of each layer i (i=1, n) on
the basis of equation (6) and table 1.
7. Compute the average lateral reaction modulus E
ti
i
of the layer
i at the mid-segments of the pile:


