2261
Influence of Ground Motion Variability on Seismic Displacement Uncertainty
Influence de la variabilité des mouvements de terrain sur l'incertitude des déplacements en régime
sismique
Strenk P.M.
Golder Associates, Inc., Reno, Nevada, USA
Wartman J.
University of Washington, Department of Civil and
Environmental Engineering, Seattle, Washington, USA
ABSTRACT: A series of probabilistic seismic displacement analyses were performed to understand how material property variability
coupled with systematic changes in the type and complexity of ground motion variability affect the displacement prediction
uncertainty of the Newmark rigid-block method.
RÉSUMÉ: Une série d'analyses probabilistes des déplacements en régime sismique ont été effectués pour comprendre comment les
variations des propriétés des matériaux couplées avec le type et la complexité des variabilités des mouvements de terrain affectent les
incertitudes dans les prédictions de déplacement avec la Méthode des blocs rigides de Newmark.
KEYWORDS: Newmark, displacement, seismic, probabilistic, ground motion, variability, uncertainty, Monte Carlo
1 INTRODUCTION
Seismic slope deformation methods are used to make
predictions of earthquake-induced permanent displacements in
natural slopes and man-made dams and embankments. The
predictive capability of well-established methods such as rigid-
block (Newmark 1965) and decoupled (Makdisi and Seed 1978)
procedures, however, are often associated with a high-degree of
uncertainty which is a consequence of both parametric and
modeling sources of variability.
Parametric variability
describes a method’s sensitivity to the range of input parameters
(e.g., shear strength, groundwater and earthquake ground
motions) and is a function of the number of input parameters as
well as the amount of variability in each parameter. Modeling
variability is related to how well the method captures the actual
physical mechanism of seismic-induced deformation when all
input parameters are fully known. Although the majority of
deformation-based method available today have a common
conceptual origin in the sliding-block model proposed by
Newmark (1965), differences in their analytical formulation,
procedural structure, underlying assumptions and mathematical
or regression functional form can result in different predictive
capabilities and sensitivities to parametric variability (Strenk
and Wartman 2011).
In seismic slope deformation analyses, parametric variability
comes from parameters characterizing the seismic demand
(earthquake ground motions) and those characterizing the
slope’s seismic resistance (represented by the seismic yield
coefficient,
k
y
which is a function of the slope geometry, shear
strength and groundwater conditions). In a probabilistic
framework, the interplay between these two sources of
parametric variability can make evaluating their relative
contributions to total displacement uncertainty a difficult task.
The main focus of this study is to examine how ground motion
variability influences the prediction uncertainty of the Newmark
(1965) rigid-block method. To that end, a series of probabilistic
seismic slope deformation analyses were performed on an
idealized slope for a scenario earthquake event. Displacement
uncertainty was quantified for several scenarios designed with
increased levels of ground motion variability. Variability in the
seismic resistance of the slope was also included. In this
approach, realistic levels of variability in both seismic
resistance and demand are systematically changed to evaluate
their collective effect on displacement prediction uncertainty.
2 PROBABILISTIC DISPLACEMENT ANALYSIS
Performing a rigid-block analysis consists of the following
steps: (1) a limit-equilibrium pseudostatic slope stability
analysis to compute
k
y
; and (2) characterization of the
earthquake-induced shaking at the site. The seismic yield
coefficient represents the minimum acceleration required to
initiate down-slope displacement of a slide mass. In the rigid-
block method, earthquake shaking is characterized by
acceleration time-histories that represent a rock outcropping
condition which is consistent with the concept of slide mass
rigidity assumed by Newmark (1965). Each of these analyses
was implemented in a probabilistic framework using Monte-
Carlo simulation. All simulations were performed for 1000
iterations using Latin-Hypercube sampling of the input
distributions.
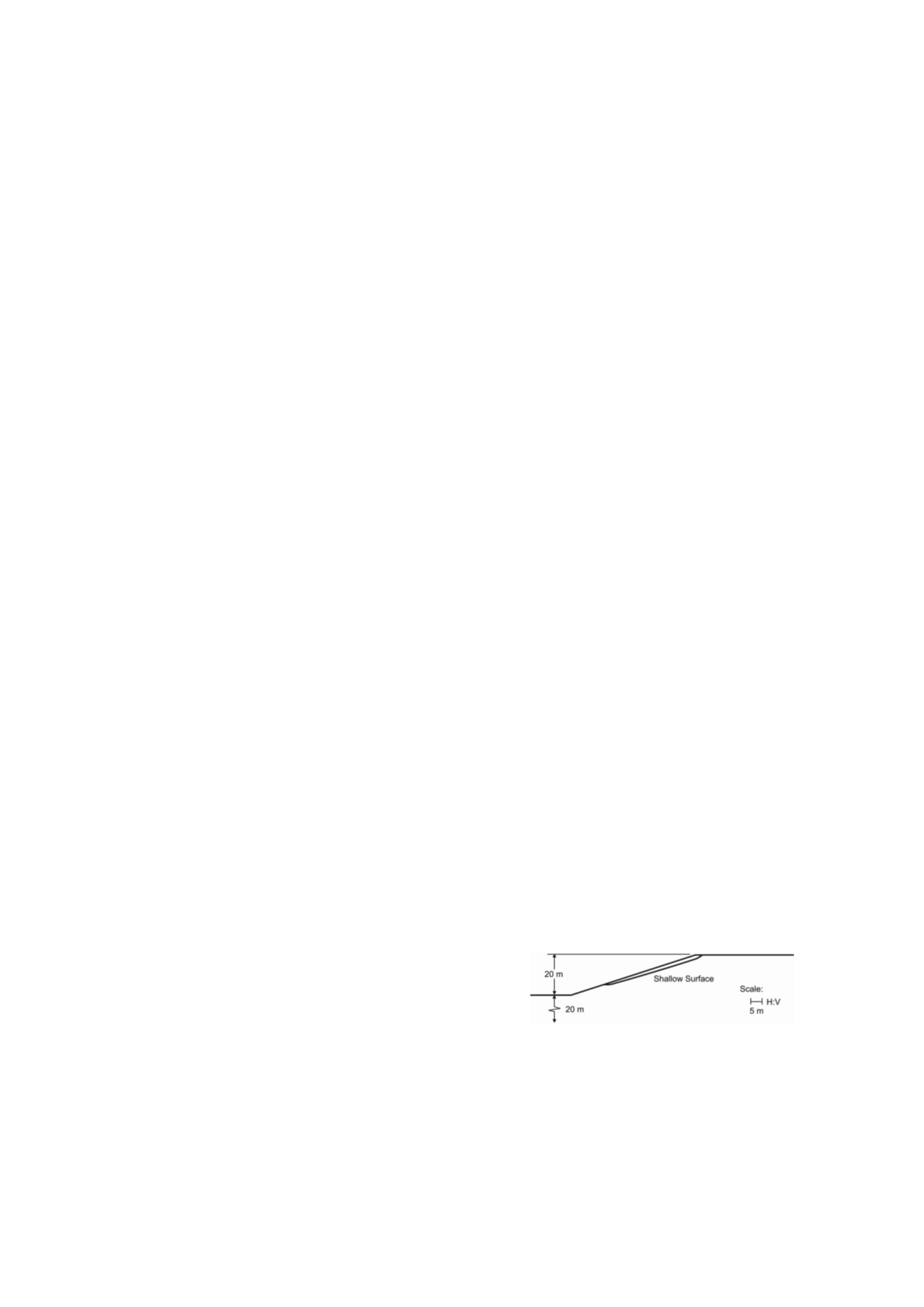
The idealized slope model has a height of 20 m with a slope
face inclined at an angle of 18 degrees. The failure surface
shown in Figure 1 is intended to represent a first-time, shallow
translational landslide. Shear strength of the landslide material
(unit weight,
= 20 kN/m
3
) was assumed to be controlled by the
peak friction angle (
'
peak
) only.
Figure 1. Cross-section of the idealized slope model with a shallow
failure surface (maximum thickness of 2 m).
The scenario earthquake used for this analysis is the 1994
Northridge event (moment magnitude,
M
w
= 6.7), in California,
USA. The slope was assumed to be located 28 km to the
northwest of the epicenter. Based on the assumed site location,
acceleration time histories were selected from recording stations
that recorded the Northridge event. Four stations were selected:
(1) Lake Hughes 12A (LHA); (2) Castaic-Old Ridge Road
(ORR); (3) Vasquez Rock Park (VAS); and (4) Newhall-West


