1265
Technical Committee 202 /
Comité technique 202
Proceedings of the 18
th
International Conference on Soil Mechanics and Geotechnical Engineering, Paris 2013
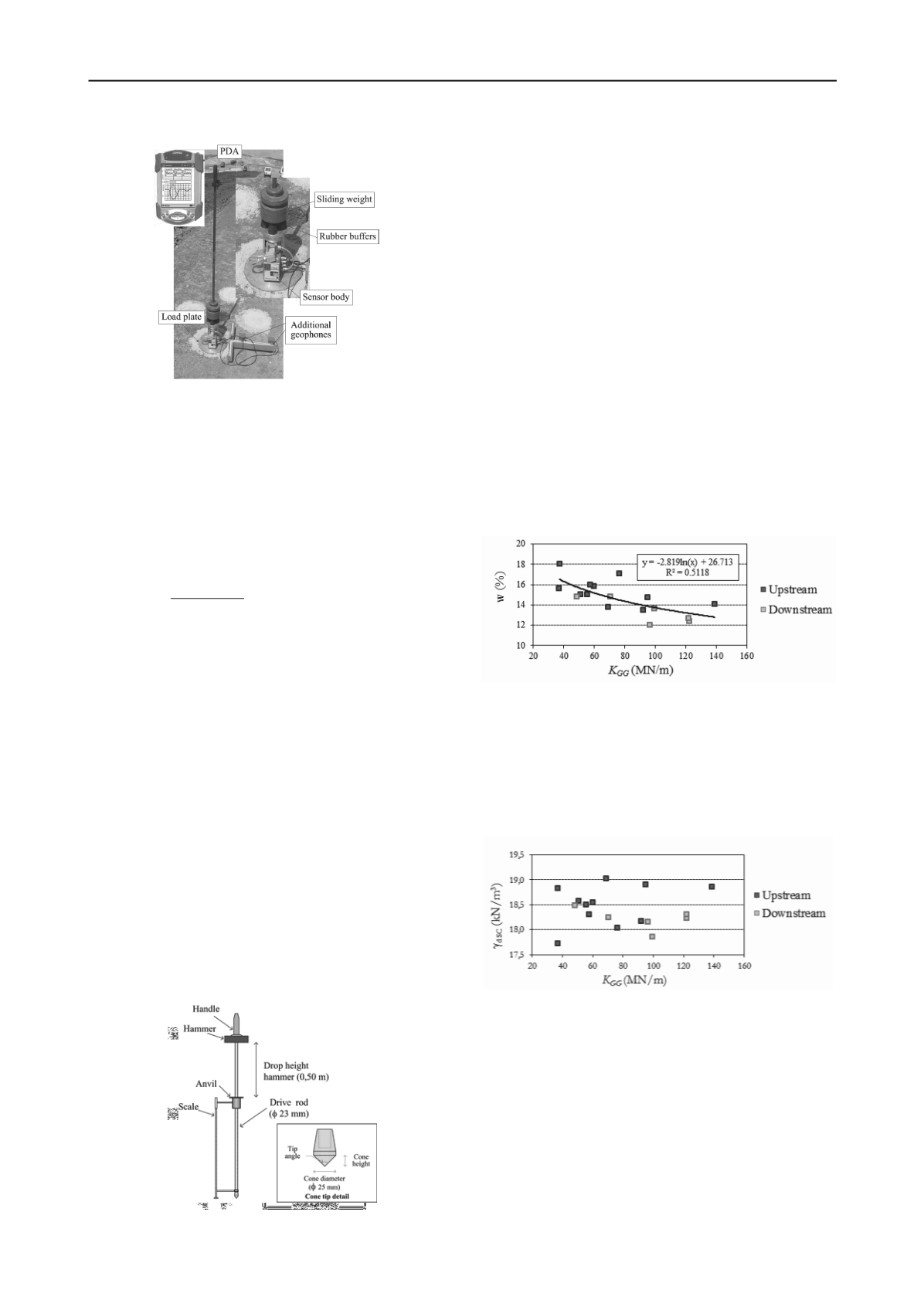
Figure 6. Prima 100 P-FWD device.
The device measures both force and deflection. The software
enables the selection of test setup and to visualize and save the
test results. Time histories and peak values of load and
deflection are displayed in a hand-held computer (PDA). The
peak values of load and deflection allow determining the elastic
stiffness modulus,
E
P-FWD
. The equation used to determine
E
P-FWD
is based on the Boussinesq’s equation. It corresponds to
calculating the surface modulus of a layered material under a
uniform circular load of radius R, assuming an uniform
Poisson’s ratio:
c
FWD P
R
f
E
2
1
(2)
where
f
is the stress distribution factor, assumed 2.0 (flexible
plate),
ν
is th
e Poisson’s ratio, assumed 0.35
,
is the (peak)
impact stress under the loading plate (kPa),
R
is the P-FWD
loading plate radius (150 mm) and
δ
c
is the (1
st
peak) P-FWD
deflection (
m).
Initially, a 300 mm diameter loading plate, four buffers, a 15
kg falling mass and a 0.8 m drop height were adopted. This
configuration proved to be inadequate due to the excessive
energy involved which caused the apparatus to detach from the
ground after impact. It was observed that this affected the
accuracy of the deflection measurement. The experimental setup
was then changed to a 10 kg falling mass and a 0.4 m drop
height, which proved to be adequate in terms of contact and
reading accuracy (Conde
et al
., 2009). With this setup the
equipment applies a contact pressure between 85 and 100 kPa.
2.6
DCP testing
Dynamic cone penetrometer (DCP) allows a simple, fast, and
economical usage and provides continuous measurement of the
penetration resistance of embankment or pavement layers. The
DCP consists of a steel rod with a cone tip at the end. In this
study a light weight configuration was used, i.e. with a 10 kg
hammer, with a falling height of 50 cm (Figure 7).
Figure 7. DCP device.
In this study DCP testing was performed according to
EN ISO 22476-2 standard.
The readings were taken
continuously through the compaction layer depth, i.e. along 40
cm, and recorded every 10 cm. Based on the total number of
blows required to drive the penetrometer through the layer, the
average penetration rate at each 10 cm penetration PN10
(mm/blow) or the cumulative number of blows N
10
were was
calculated.
3 RESULTS ANALYSIS
To evaluate the performance of these devices as tools for the
compaction control of embankment layer, the sensitivity of the
results to variations in water content and in dry density of
geomaterials determined by traditional methods was assessed.
3.1
K
GG
results
Figure 8 shows the correlation between water content (w) and
K
GG
. The chart shows some scattering about the adjusted
negative exponential trend which limits the quality of the
adjustement. A significant increase of the geogauge stiffness
with decreasing water content may be inferred from the data
both in the upstream and in the downstream shells.
Figure 8. Relation between soil stiffness, k
GG
, and water content, w.
Regarding the dependence of
K
GG
on the
in situ
dry density,
the results in Table 2 present minor variations of the relative
compaction, i.e. of
d SC
,
thus making the correlation analysis
dificult. Conde
et al
. (2010) analyzed these results concluding
that small and erratic sensitivity of stiffness values determined
by geogauge occurred with only relatively small variations in
dry density (Figure 9).
Figure 9. Soil stiffness,
k
GG
, and dry density,
d SC
, results.
The joint consideration of both results seems to indicate that
soil stiffness is only a reliable predictor of the water content
variation. This sensitivity of stiffness to changes in water
content were also observed by Abu-Farsakh
et al.
(2004) in a
study conducted on fine soils (silt, sandy clay and clay).
3.2
E
P-FWD
results
Figure 10 shows the relationship between the elastic stiffness
modulus (
E
P-FWD
) and the
in situ
water content (w). While the
adjusted trend is again of the negative exponential type, a
smaller scatter is now observed in comparison with that of the
geogauge results.
100 P-FWD device.
measures both force and deflection. The software enables the selection of test setup and to visualize and save
s. Time histories and peak values of load and deflection are displayed in a hand-held computer (PDA). The peak
and deflection allow determining the elastic stiffness modulus,
E
P-FWD
. The equation used to determine
E
P-FWD
he Boussinesq’s equation. It corresponds to calculating the surface modulus of a lay red m terial under a
lar load of radius R, assuming an uniform Poisson’s ratio:
c
R
f
2
1
(1)
stress distribution factor, assumed 2.0 (flexible plate),
ν
is the Poisson’s ratio, assumed 0.35,
is the (peak)
under the loading plate (kPa),
R
is the P-FWD loading plate radius (150 mm) and
δ
c
is the (1
st
peak) P-FWD
).
300 mm diameter loading plate, four buffers, a 15 kg falling mass and a 0.8 m drop height were adopted. This
proved to be inadequate due to the excessive energy involved which caused the apparatus to detach from the
mpact. It was observed that this affected the accuracy of the deflection measurement. The experimental setup
nged to a 10 kg falling mass and a 0.4 m drop height, which proved to be adequate in terms of contact and
acy (Conde
et al
., 2009). With this setup the equipment applies a contact pressure between 85 and 100 kPa.
sting
e penetrometer (DCP) allows a simple, fast, and economical usage and provides continuous measurement of the
sistance of embankment or pavement layers. The DCP consists of a steel rod with a cone tip at the end. In this
eight configuration was used, i.e. with a 10 kg hammer, with a falling height of 50 cm (Figure 2).
device.
dy DCP testing was performed according to EN ISO 22476-2 standard. The readings were taken continuously
mpaction layer depth, i.e. along 40 cm, and recorded every 10 cm. Based on the total number of blows required
enetrometer through the layer, the average penetration rate at each 10 cm penetration PN10 (mm/blow) or the
mber of blows N
10
were was calculated.


