2749
Technical Committee 212 /
Comité technique 212
Figure 5. Increment stiffness plot of records from Levels 1 to 3,
Pile TP02
1.3
Load distribution along the pile shafts
The evaluated stiffness (EA) values were used to convert the
strain measurements to load. Figure 6 shows the so-evaluated
load distributions. The shaft resistances shown along the upper
about 30 m for both piles were not fully mobilized.
Figure 6. Load Distributions with Approximate Head-down
Curve of Piles TP01 and TP02
As shown in Figure 1, the soil at the depth of SG1 to SG3
was a firm clay layer. It is a reasonable expectation that the
load distributions would have shown a gentle shape with a
decreasing slope toward depth, reflecting increasing shaft
resistance as SPT N-indices increase with depth. That this is
not the case is considered due to varying effect of the slurry
filter cake and, possibly varying pile cross section.
Assuming that the shaft resistance would show a uniform
proportionality to effective overburden stress, Figure 6 shows
the actual and approximate equivalent head-down load-
distribution curves. The latter are obtained by an effective stress
calculation for ß-coefficient of 0.2 to 18.5 m depth and 0.3
below this depth to the pile toes. The effective stress analysis
indicates the toe resistance and total shaft resistance of Pile
TP01 are 3,723 and 4,088 KN, and of Pile TP02 are 4,495 KN
and 4,932 KN, respectively.
1.4
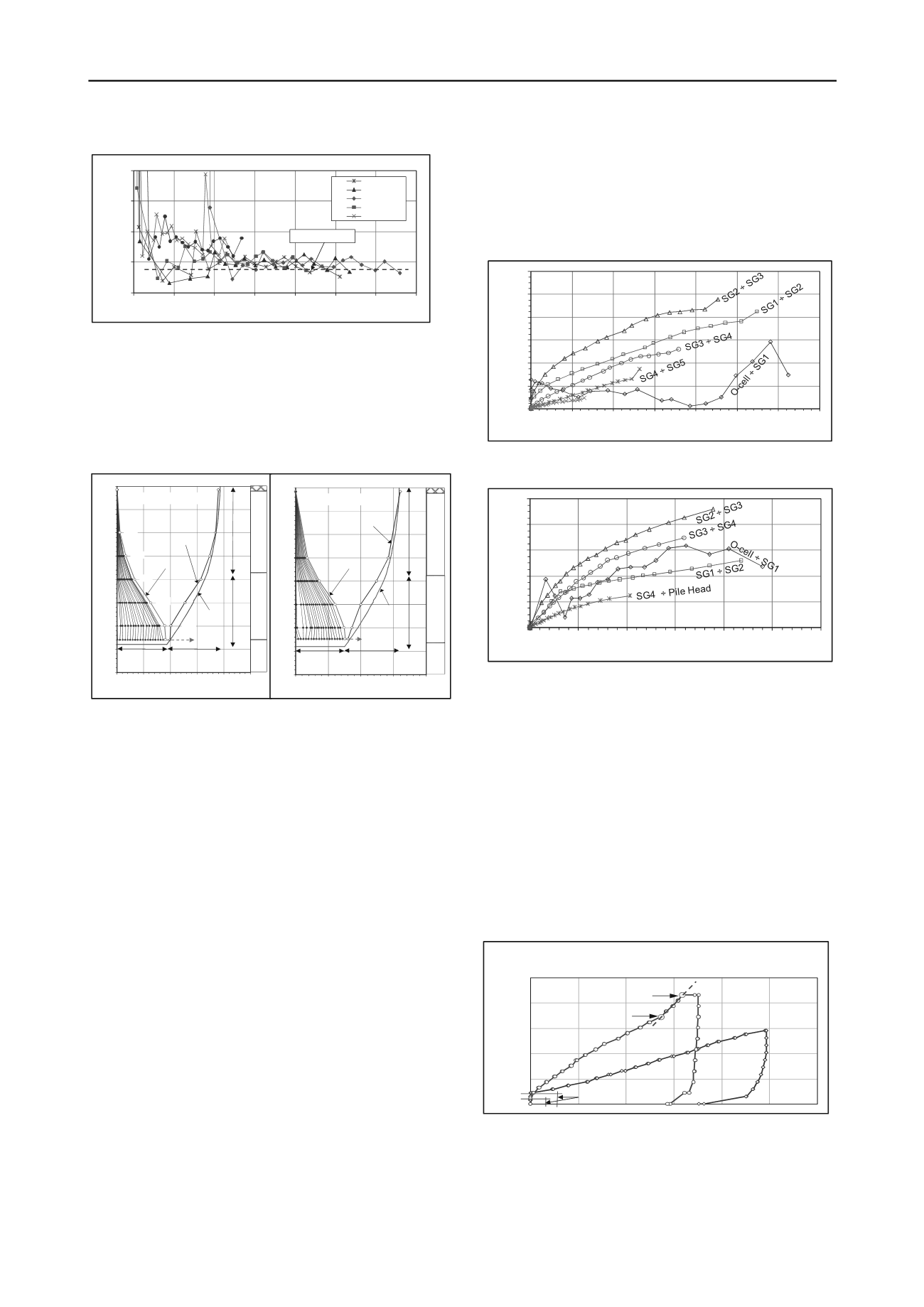
Unit stress versus movement for shaft resistances and pile
toes
The average unit shaft shear resistance between the gage levels
can be determined as the difference in evaluated strain-gage
load divided by the surface area between the gage levels.
Figures 8 and 9 show those values plotted against movements
for the particular gage levels. The maximum values of the unit
shaft resistance evaluated between the O-cell and SG1 for both
test piles showed the average unit shaft resistances of about
60 kPa. Because of strain-softening, the shear resistances were
smaller than expected. Moreover, the resistances from SG1
through SG3 are smaller than between SG2 and SG3 for both
piles.. It is probable that the construction process left a filter
cake between the concrete and the soil and, therefore, the shear
movement occurred in the filter cake. The curves also show
that, for both piles, ultimate shaft resistance along the length
above the O-cell level has not occurred excepting for the curves
from O-cell to SG1. The uppermost gage level movements and
loads were too small to produce meaningful curves in pile. The
records of unit shear resistance between SG4 and SG5 in Pile
TP02 were unreliable and are therefore omitted.
0
20
40
60
80
0
50
100
150
200
250
300
350
SG - 1A
SG - 1B
SG - 2A
SG - 2B
SG - 3A
INCREMENTAL STIFFNESS AE (GN)
STRAIN(μS)
AE = 17 (GN)
Figure 8. Average shear resistance between gage levels of Pile
TP01
0
20
40
60
80
100
120
0.0
0.5
1.0
1.5
2.0
2.5
3.0
3.5
MOVEMENT (mm)
AVERAGE SHEAR (KPa)
SG5 ÷ Pile Head
0
5
10
15
20
25
30
35
40
0 2,000 4,000 6,000 8,000 10,000
LOAD(KN)
DEPTH (m)
Measured
Distributio
n
Figure 9. Average shear resistance between gage levels of Pile
TP02
Figure 10 shows stresses applied to the pile toe versus the
pile toe movements divided by the pile diameter in percent. The
stresses are determined by dividing the measured load at O-cells
with the nominal shaft areas below O-cell. The maximum toe
movements were about 3 and 5 mm, respectively. The
increasing stiffness measured for Pile TP01 is considered due to
the debris at the pile toe having become compressed.
For both piles, the pile toe stress-movement response was
essentially linear and showed no tendency toward an ultimate
resistance. It is obvious that the pile toe response is not
representative for pile toes placed in weathered sandstone or in
sand. Similar observation for large diameter bored piles is
reported by (Fellenius and Nguyen 2013).
Figure 10. Stress-% downward movement curve of Piles TP01
and TP02
1.5
Back-analysis to response long-term settlements of piles
The back-analysis of two tested piles was performed by “The
Unified Pile Design” method (Fellenius 1984; 1988; 2011).
O-cell
#4
#3
#2
#1
#5
SILTY
SAND
FIRM
CLAY
SAND
STONE
ExistingGroundSurface
Rt= 3,723KN Rs= 4,088KN
TP01 (D800)
Equivalent
Head-down
Distribution
Approximate
Head-down
Distribution
0
5
10
15
20
25
30
35
40
0
3,000 6,000 9,000 12,000
LOAD(KN)
DEPTH (m)
0
20
40
60
80
100
0.0
1.5
3.0
4.5
6.0
7.5
9.0
MOVEMENT (mm)
AVERAGE SHEAR (KPa)
O-cell
ExistingGroundSurface
SILTY
SAND
#4
#3
#2
#1
FIRM
CLAY
SAND
STONE
#5
Measured
Distribution
TP02 (D1000)
Equivalent
Head-down
Distribution
Rt= 4,495KN
Approximate
Head-down
Distribution
Rs= 4,932KN
0.0
0.5
1.0
1.5
2.0
2.5
0
1
2
3
4
5
6
MOVEMENT/DIAMETER (%)
TP01-D800
TP02-D1000
NORMALIZED TOE STRESS (MPa)
Breaking Bonds


