3108
Proceedings of the 18
th
International Conference on Soil Mechanics and Geotechnical Engineering, Paris 2013
Table 1: Tensile properties of the model geosynthetics
Table 2: Conversion of characteristic strength properties model/prototype
(Index k: characteristic)
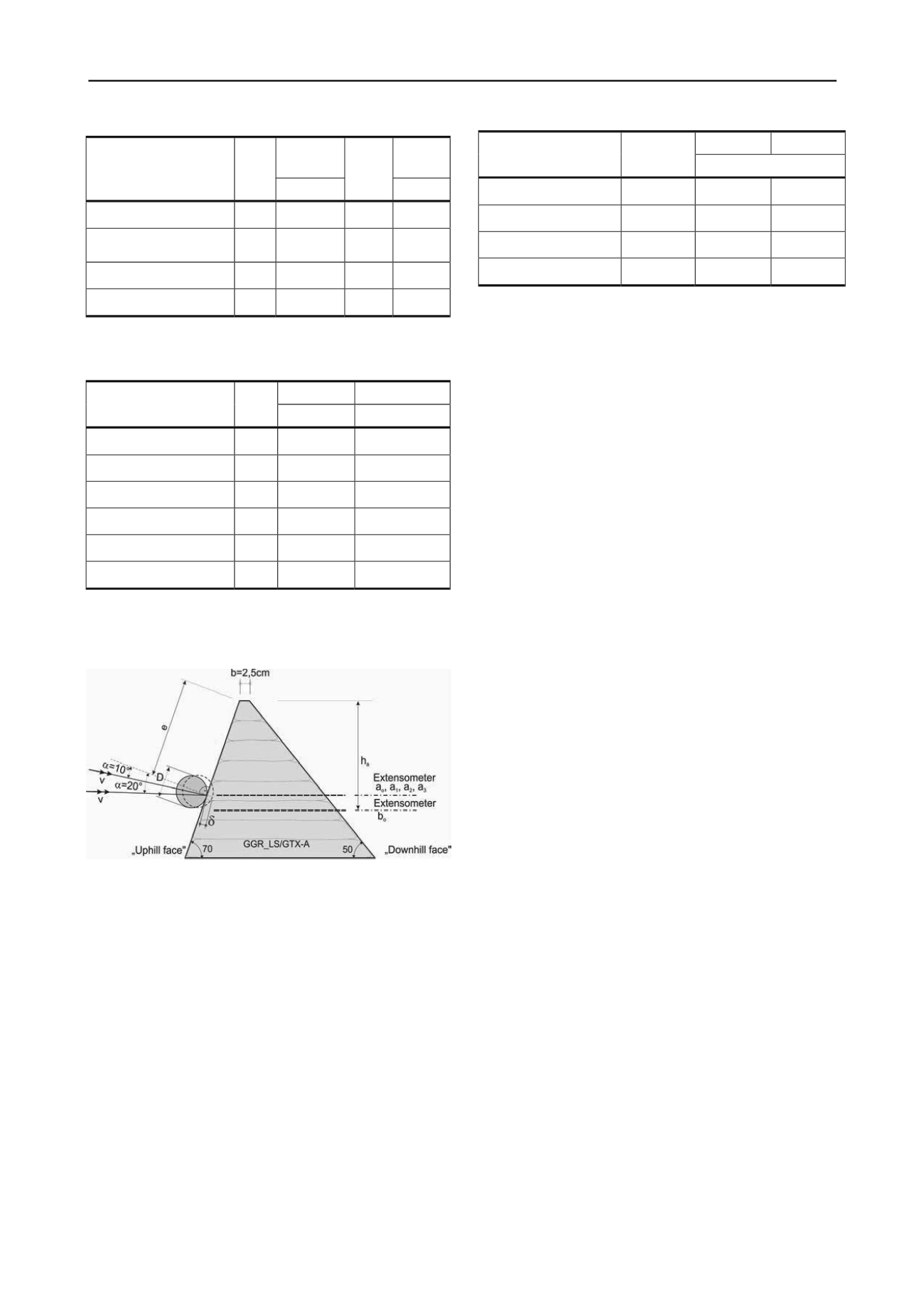
Figure 1. Cross section with extensometers
2.3
Model embankments with geosynthetics
The model embankment was reinforced with geosynthetics
GGR_LS and GTX_A supplied by NAUE (Table 1).
If the model laws are observed, then a line load in this model has a
transfer factor
² = 33² = 1089 [-]. The characteristic values given
in Table 2 are derived using this and equate to the full-scale values
characteristic for the prototype.at a scale of 1:1.
The effective tensile strengths in the model are obtained using
a factor of A2 = 1.2 for installation damage resulting from sub-
base placement and compaction, as are the strengths FP
,d
relevant
for the prototype after application of all reduction factors e.g.
installation damage (Table 3).
2.4
Model embankments with stacked-rock facing
In some tests, a rip rap facing was used on the uphill face of the
embankment (Figure 2).
F
P,d
J
P,d@5%
Property
Unit
Geogrid
Tensile strength md
kN/m
226.9
4538
@ Extension
, md
%
5
-
Tensile strength cmd
kN/m
127.1
2542
@ Extension
, cmd
%
5
-
Table 3: Required values for reinforcement of the prototype
(Index d: design)
3 BASIS FOR DESIGN MODEL
3.1
Evaluation using relative impact energy and penetration
depth
In the model tests, the size and shape of the failure body created
by the sphere were recorded. With the help of a non-dimensional
evaluation of the test results, diagrams were drawn up to enable
the results to be transferred to the full-scale situation, Hofmann &
Mölk 0.
For the evaluation, an activated soil body was defined near the
embankment crest (Figure 3). A non-dimensional depiction of the
results is shown in Figure 4. The relative impact energy E* was
introduced and plotted against the non-dimensional value δ/b,
where δ is the depth of penetration of the sphere into the
embankment and b is the crest width. The upper extensometers (a
0,
a
1,
a
2,
a
3
) were positioned at the mid-point of the sphere in the axis
of the embankment; one extensometer was positioned at the level
of the bottom of the sphere. The following variables are
introduced:
E*= E / (γ * A
a
* D * h
a
) (-)
(1)
E = m v²/2 (Joule)
(2)
γ= ρ * g (N/m³)
(3)
A
a
= (b+c)/2 * h
a
(activated area) (m²)
(4)
where E* is the relative impact energy, m is the mass of the sphere
in kg, v its velocity in m/s (v
1
;v
2
;v
3
), ρ the soil density in kg/m³, g
the gravitational acceleration in m/s², D the diameter of the sphere
in m, h
a
the activated height in m and b the crest width in m.
3.2 Lessons learned from the tests
3.2.1
Lessons from the model tests for all construction types
In the vicinity of the impact, the body of the
embankment undergoes significant compaction, and the
crest is displaced upwards.
The slimmer the construction, the larger the failure body
on the downhill face of the embankment.
A comparison of the different model tests clearly shows
the more pronounced elasto-plastic behaviour of the
embankment reinforced with geogrids compared with
the unreinforced case (Figure 4).
3.2.2 Lessons from trials without rip rap facing and without
geosynthetics
A comparison shows how the deformations increase
significantly from the lowest point of the sphere
upwards. Observation of the model tests shows that a
freeboard of at least twice the diameter D of the sphere
F
M,k
GGR_LS
F
P,k
Property
Unit
Geogrid
Geogrid
Tensile strength md
kN/m
0.25
1089
272.3
Extension
, md
%
5
-
5
Tensile strength cmd
kN/m
0.14
1089
152.5
Extension
, cmd
%
5
-
5
GGR_LS
GTX_A
Property
Unit
Geogrid
Nonwoven
Mass per unit area
g/m²
28
39
Thickness
mm
0.24
0.25
Tensile strength md
kN/m
0.25
0.36
Extension
, md
%
5
16
Tensile strength cmd
kN/m
0.14
0.09
Extension
, cmd
%
5
55


