1006
Proceedings of the 18
th
International Conference on Soil Mechanics and Geotechnical Engineering, Paris 2013
Figure 5. Experimental probability transition of a particle
d
i
in to a
particle
d
j
.
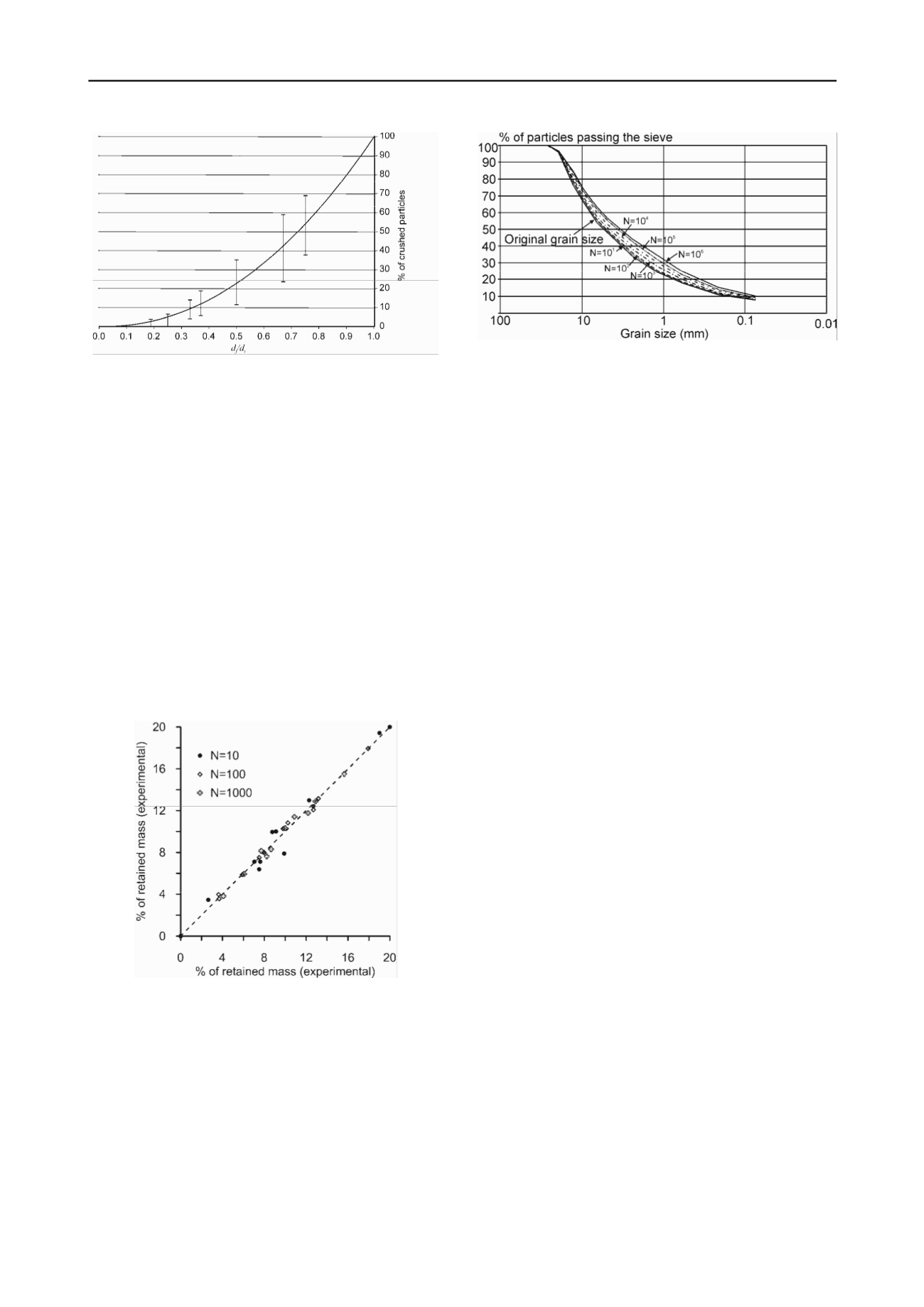
Figure 7. Evolution of the grain size distribution for different number of
loading cycles.
4 CONCLUSION
Elements
p
ij
of the transition probability matrix can be
calculated as follows:
) , (
j
i
f
ij
dd Beta
p p
(19)
Finally the evolution of the grain size distribution of a
granular mixture results from the product of the transition
probability matrix (transposed) and the grain size distribution
before the loading cycle:
This paper presents a new model to assess the process of
changes of the granular material properties as fracturing and
abrasion occur as a result of cyclic loading. The model has
shown that it can accurately predict the deterioration process of
unbound granular materials subject to cyclic loading. This
analytical model is based on the theory of poly-disperse
mictures and therefore calculations up to high number of
loading cycles can be performed without the difficulties of
discrete element modelling. The results show very good
agrement with the experimental tests illustrating the possibilities
of this new model.
1
Ni
T
Ni
y
y
(20)
5 REFERENCES
3 RESULTS
To verify the predictions of the model three different granular
materials were tested in a gyratory compactor. This apparatus
reproduce the stress rotation during field compaction of granular
layers. Compaction was performed to different number of
loading cycles and then the grain size distribution was analyzed.
Bolton M D (1999). The role of micro-mechanics in soil mechanics
Proceedings of the international workshop on soil crushability.
Yamaguchi, Japan, 58-82
Bolton M D, McDowell G R Clastic mechanics IUTAM Symposium on
Mechanics of Granular and Porous Materials. Cambridge, 35-46
De Larrard F (2000) Compacite et homogeneite des melanges
granulaires. In: L. C. d. P. e. Chaussées (ed) Structures Granulaires
et Formulation des Betons, 1st edn. LCPC.
Feda J (2002) Notes on the effect of grain crushing on the granular soil
behaviour. Engineering Geology, 63(1-2): 93-98
Hagerty M M, Hite D R, Ullrich C R, Hagerty D J (1993) One-
dimensional high-pressure com-pression of granular media. Journal
of Geotechnical Engineering, 199(1): 1-18
Hardin B O (1985) Crushing of soil particles. Journal of Geotechnical
Engineering, 111(10): 1177-1192
Lade P V, Yamamuro J A, Bopp P A (1996) Significance of Particle
Crushing in Granular Ma-terials. Journal of Geotechnical
Engineering 122(4): 309-316
Lee, D. M. (1992). "The angles of friction of granular fills," Ph.D.
dissertation, University of Cambridge.
Lobo-Guerrero S (2006) Evaluation of crushing in granular materials
using the discrete element method and fractal theory. University of
Pittsburgh, Pittsburgh, PA.
McDowell G R, Bolton M D (1998) On the micromechanics of
crushable aggregates. Géotechnique, 48(5): 667-679
Nakata Y, Hyde F L, Hyodo M, Murata H (1999) A probabilistic
approach to sand particle crushing in the triaxial test. Géotechnique,
49(5): 567-583
Figure 6. Comparison between experimental and model results.
Figure 6 present a comparison of the experimental and
numerical tests for one of the tested materials. This figure
shows the mass of particles retained in each sieve size. Each
point in the figures corresponds to a particle size at the end of a
specific number of loading cycles. These figures show the very
good agreement between the model and the experimental data.
Nakata Y, Hyodo M, Hyde F L, Kato Y, Murata H (2001) Microscopic
particle crushing of sand subjected to high pressure one-
dimensional compresion. soils and Foundations, 41(1): 69-82
Ocampo M (2009) Fracturamiento de partículas en materiales
granulares sometidos a cargas cíclicas con rotación de esfuerzos.
Universidad de Los Andes, Bogotá D.C.
Figure 7 represent an extrapolation of the results of the
model up to a million of loading cycles showing the capacity of
this analytical model to calculate the evolution of grain size
distribution when a high number of cycles are applied to the
material.
Yamamuro J A, Lade P V (1996) Drained Sand Behavior in
Axisymmetric Tests at High Pres-sures. Journal of Geotechnical
Engineering, 122(2): 109-119


