994
Proceedings of the 18
th
International Conference on Soil Mechanics and Geotechnical Engineering, Paris 2013
Quantitative analysis of the processed images reveals that the
particle size distribution evolves in rather a surprising manner,
and an attempt is made to correlate this to analytical creep
parameters coming from Yin (1999). This is a nice example of
analysis in which measurements at the micro scale are related to
a macroscopic model. Further work might benefit from more
robust image analysis techniques.
The study presented by
Minder & Puzrin (2013)
is extremely
interesting and very well suited to this session. In order to
achieve a
macroscopic
objective (increasing the permeability of
a clay soil), the Authors develop an innovative experimental
technique to modify the
microstructure
of the soil by using
cation exchange. More precisely, the highly selective and
strongly exchanging organic cation guanidinium was used to
stabilize the interlayer distance between clay platelets. The
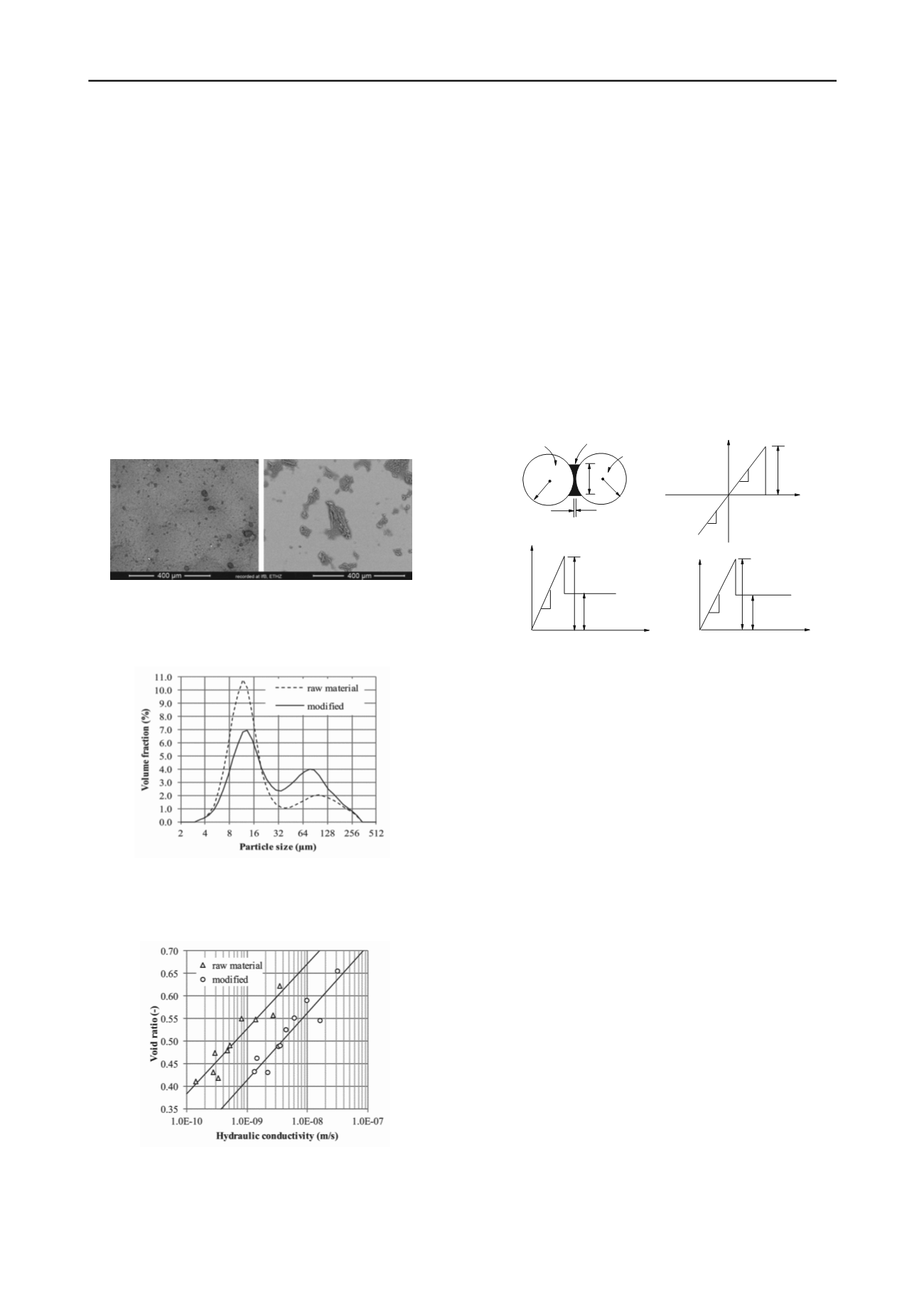
effectiveness of this chemical treatment is characterized at the
micro scale using SEM (see Fig. 5) and laser diffraction (see
Fig. 6) as well as at the macro scale, where the improvement
appears both in terms of increased permeability (see Fig. 7) and
enhanced shear strength.
Figure 5. SEM-images of bentonite grains after washing in suspension
with demineralised water. The calcium form remains finely dispersed
(left), whereas the exposure to guanidinium ions (right) leads to the
formation aggregates
(Minder & Puzrin 2013)
.
Figure 6. Bimodal particle size distribution measured with laser
diffraction. The volume fraction of the larger mode (aggregates) is
significantly increased by the treatment
(Minder & Puzrin 2013)
.
Figure 7. Decrease of hydraulic conductivity during sample
compaction (including log-linear regression) of quartz-bentonite
mixtures. For identical void ratio the modified soil is constantly about
one order of magnitude more permeable
(Minder & Puzrin 2013)
.
3.3
Learning mechanics from DEM
In the study by
Jiang et al. (2013)
, DEM is used to describe at
the particle level the mechanics of sand containing methane
hydrates. In fact, the presence of methane hydrates in deep sea
beds significantly alters the mechanical properties of the host
sand material, because methane hydrates act as a bond between
particles. The study by
Jiang et al. (2013)
introduces a
simplified contact model (see Fig. 8), which was experimentally
calibrated in the laboratory. The bond failure criterion is directly
linked to the strength of methane hydrates, which depends on
temperature, mean normal stress, density and methane hydrate
saturation of the sand. The results of DEM simulations are
compared to experimental results for the case of plane strain
compression of a methane hydrate bearing sand; the results
seem to show that although highly simplified, this model
qualitatively captures the mechanical effects of cementation at
the macro scale.
soil grain
tension
(d)
(c)
(b)
(a)
M
u
s
F
s
F
n
u
n
R
rb
Residual
strength
soil grain hydrate
B
R
nb
1
K
n
K
n
1
R
sb
1
K
s
Residual
moment
1
K
r
compression
R
1
R
2
t
Figure 8. Schematic illustration of (a) MH bonded soil grains and its
response: (b) normal contact force
F
n
against overlap
u
n
;
(c) shear
contact force
F
s
against relative shear displacement
u
s
; and (d) contact
moment
M
against relative rotation
(
Jiang et al. 2013
).
Ground loss at the head of tunnels and in mining operations is a
classical geotechnical problem with important implications for
infrastructure development in urban settings. It has been studied
at the laboratory scale for a long time; see for example the now
classical trapdoor experiment by Terzaghi (1936). The paper by
Kikkawa et al. (2013)
reports a 3D DEM study of trapdoor
unloading and gravity flow of granular material. The geometry
of the problem studied is the same of the trapdoor experiments
previously performed by Kikumoto & Kishida (2003) – see
Fig.9a. Although the results of the DEM simulations agree well
with the measurements from the actual experiments in some
respects (for example, the vertical stress on the trapdoor when it
is moved downward), DEM is substantially off target in other
instances, for example in terms of the settlement of the surface
of the sand above the trapdoor, see Fig. 9b. These “major
discrepancies” are attributed by the Authors to the difference
between the actual grains of Toyoura sand (used in the
experiments) and the particles used in the DEM modeling
(which are much larger and spherical). The general lesson to be
learnt here is that the application of DEM to the analysis of
boundary value problems is not trivial, especially when one
seeks quantitative results and not only for a qualitative insight –
calibration of the model remaining a major issue.
It has been often advocated that the use of DEM in
micromechanical studies can significantly help advance our
understanding of fundamental geomechanics (
e.g
., O’Sullivan
2011). In the writer’s opinion, DEM simulations are in fact a
very useful tool for investigating the complex behavior of
particulate materials, especially in conjunction with laboratory
tests. In this respect, the study by
Ning & Evans (2013)
is of
particular interest. The Authors address the fundamental issue
of shear wave propagation in granular soil, using DEM
simulations to investigate the effects of excitation frequency,


