2988
Proceedings of the 18
th
International Conference on Soil Mechanics and Geotechnical Engineering, Paris 2013
refrigeration capacity. The reason for this increase is the
additional convective heat flow caused by the groundwater
flow. The flow velocity influences the artificial ground freezing
application twice, because the increased refrigeration capacity
has to be hold up for a longer time period to freeze the required
frost body contour and during the operating phase.
5 ACKNOWLEDGEMENTS
Figure 5 displays that the refrigeration capacity with
groundwater flow decreases with time due to an increasing frost
body. However, the refrigeration capacity for a flow velocity of
1.0 m/d and 2.0 m/d proceeds constant. This implies an
stagnating frost body growth, a steady state. Such a steady state
indicates a thermal equlibrium of the heat supplied by the
groundwater flow and extracted by the freeze pipes.
The displayed results have been gained from an ongoing
research project “Energetic saving potential for the construction
of complicated basements using the artificial ground freezing
method” funded by the Federal Office for Building and
Regional Planning (BBR). Sincere thanks are given to the BBR.
Furthermore the Wayss & Freytag Ingenieurbau AG, the Züblin
Spezialtiefbau GmbH and the Deilmann-Haniel Shaft Sinking
GmbH deserve our thanks for the financial support. Our thanks
also go to the Geophysica Beratungsgesellschaft mbH for their
active support in the further development of SHEMAT.
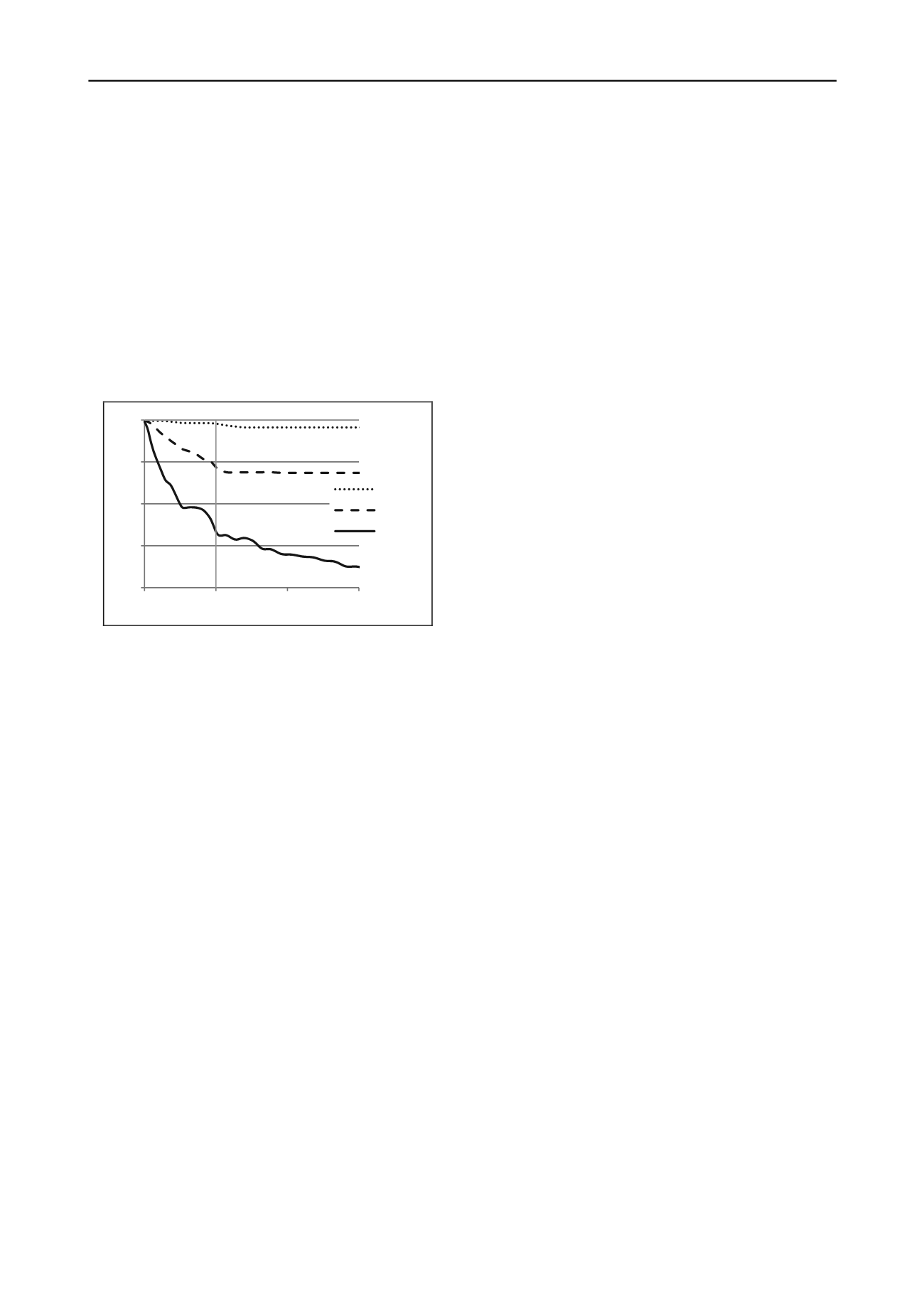
Monitoring points in the soil around the freeze pipe also
indicating a stagnation in temperature course confirm this
assumption (see Figure 6).
6 REFERENCES
-10
-5
0
5
10
0
100
200
300
Temperature [°C]
Time [h]
v = 2.0 m/d
v = 1.0 m/d
v = 0
Baehr S. and Stephan K. 2006.
Wärme- und Stoffübertragung
. Springer,
Berlin.
Baier Ch. 2009.
Thermisch-hydraulische Simulationen zur Optimierung
von Vereisungsmaßnahmen im Tunnelbau unter Einfluss einer
Grundwasserströmung
. Dissertation, Lehrstuhl für Geotechnik im
Bauwesen der RWTH Aachen.
Clauser C. 2003.
Numerical Simulation Of Reactive Flow in Hot
Aquifers – SHEMAT and PROCESSING SHEMAT
. Springer, New
York.
Gnielinski V. 1995.
Ein neues Berechnungsverfahren für die
Wärmeübertragung im Übergangsbereich zwischen laminarer und
turbulenter Rohrströmung
. Forschung im Ingenieurwesen, Vol.61,
No. 9, pp. 240-248.
Hellström G. 1991.
Ground heat Storage – Thermal Analyses of Duct
Storage Systems
. Ph.D. thesis, Theory. Dep. Of Mathematical
Physics, University of Lund.
Mottaghy D. and Dijkshoorn L. 2012.
Implementing an effective finite
difference formulation for borehole heat exchangers into a heat and
mass transport code
. Renewable Energy 45, pp.59-71.
Figure 6. Temperature course of a monitoring point in the upstream.
Comparing the refrigeration capacity after 100 hours, when
frost body still grows for all flow velocities, it becomes clear
that the refrigeration capacity increases about 10 % for a flow
velocity of 1.0 m/d and even about 25 % for a flow velocity of
2.0 m/d
Mottaghy D. and Rath V. 2006.
Latent heat effects in subsurface heat
transport modelling and their impact on palaeotemperature
reconstructions
. Geophysical Journal International 164, p. 234-245.
Verein Deutscher Ingenieure 2010.
VDI Heat Atlas
, 2nd ed. Springer,
New York.
Ziegler M. and Baier Ch. and Aulbach B. 2009.
Optimization of
artificial ground freezing applications for tunneling subject to
water seepage
. Safe tunneling for the city and for the environment:
proceddings ITA-AITES World Tunnel Congress, Budapest.
4 CONCLUSION
An approach for the realistic determination of the refrigeration
capacity by calculating the heat transfer processes within a
freeze pipe was presented. By separating the “freezrefcap”
module from SHEMAT and defining only two necessary
interfaces for the coupling a very fine discretization and long
computing times as a consequence can be avoided. The module
offers the opportunity to calculate the outlet temperature and as
a result the refrigeration capacity by entering the inlet
temperature and the flow rate of the refrigerant. Thus the
influence of different refrigerants on the refrigeration capacity
can be estimated by numerical simulations.
The aim of further research is to validate the “freezrefcap”
module by simulating a laboratory model test influenced by
groundwater flow. Thus quantitative statements on the outlet
temperature and the refrigeration capacity can be given for
various artificial ground freezing applications subject to water
seepage.
At this point qualitative statements already indicate that the
refrigeration capacity increases disproportionally with an
increasing flow velocity. In the further research process the
influence of the operating phase on the total refrigeration
capacity and the related energy consumption is determined. The
aim of the research project is the simulation and optimization of
artificial ground freezing applications regarding both time and
energy aspects already in the design phase.


