734
Proceedings of the 18
th
International Conference on Soil Mechanics and Geotechnical Engineering, Paris 2013
0
2
4
6
8
10 12 14 16 18
0
20
40
60
80
100
120
Nl
Frequency
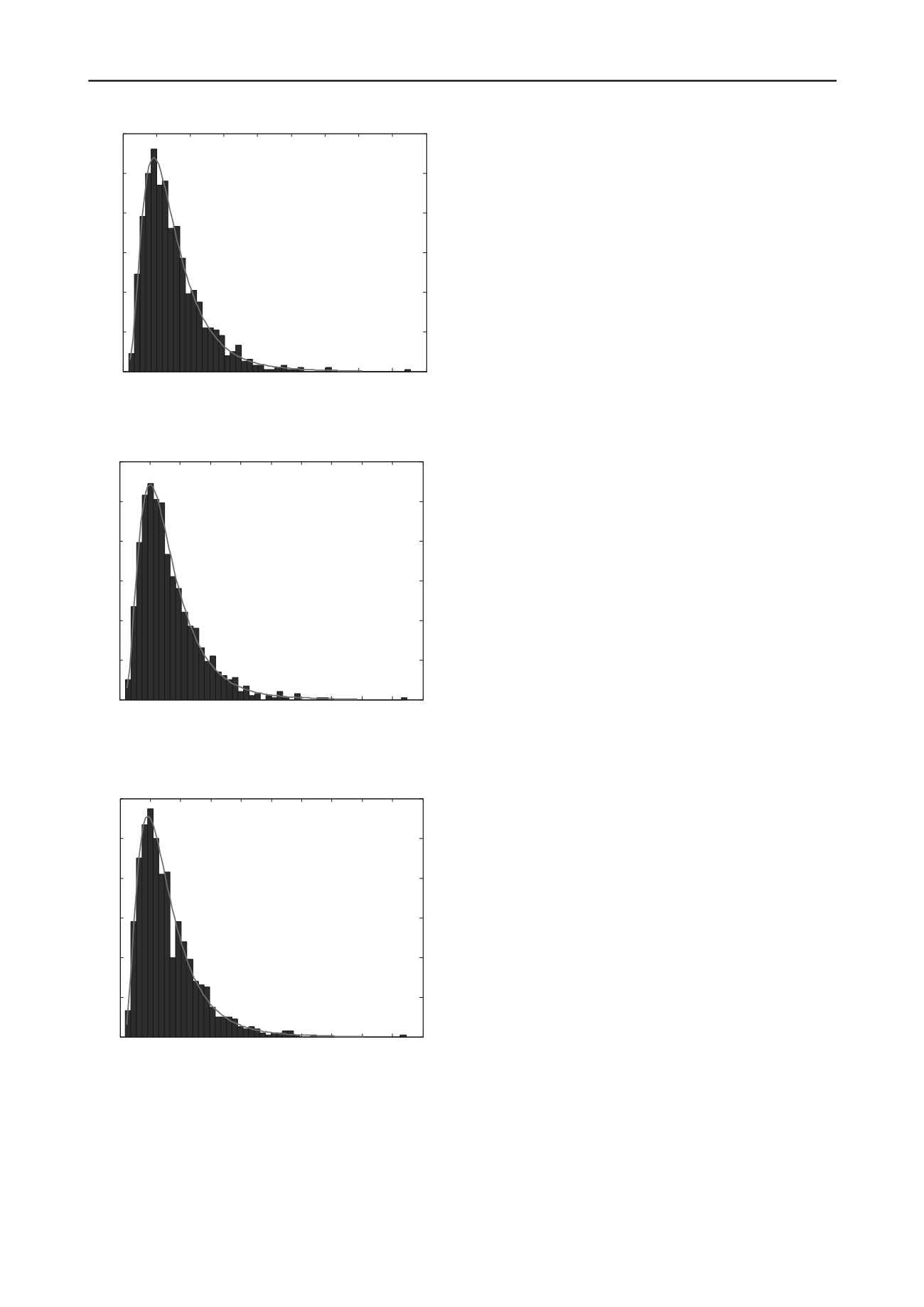
Figure 7.
Histogram and log-normal fit for the computed lower bound
bearing capacity factors when
2
u
c
and
1
u
c
V
0 2 4
6 8 10 12 14 16 18 20
0
20
40
60
80
100
120
Nu
Frequency
Figure 8.
Histogram and log-normal fit for the computed upper bound
bearing capacity factors when
2
u
c
and
1
u
c
V
0 0.2 0.4 0.6 0.8 1 1.2 1.4 1.6 1.8 2
0
20
40
60
80
100
120
Nd
Frequency
Figure 9. Histogram and log-normal fit for the difference
between the computed lower and upper bound bearing capacity
factors when
2
u
c
and
1
u
c
V
Figures 7 and 8 show histograms of lower and upper bounds
of bearing capacity factors with best-fit lognormal distributions
for the case where
2
u
c
and
1
u
c
V
. The Pearson's
coefficient of correlation between the lower and upper bounds
was found to be 0.9995, which implies a strong correlation.
This is expected since the same random field was used for both
lower and upper bound analyses. The histogram of the
difference between the lower and upper bounds is plotted in
Figure 9, which is also well fitted by a lognormal distribution.
Although nothing is known in elementary probability theory
about the distribution of the difference of two lognormals,
Figure 9 suggests the difference is a lognormally distributed
random variable, at least when the two lognormals are strong
correlated.
7 CONCLUDING REMARKS
The paper has investigated the bearing capacity factor using
both lower and upper bound limit anlysis combined with
random field theory. The mean upper bound bearing capacity
factors are always lower than the Prandtl solution using mean
soil strength. The main conclusion is that by implicitly
assuming an infinite spatial correlation in traditional first order
probabilistic analysis (e.g., First Order Second Moment and
First Order Reliability Method) may overestimate the mean
bearing capacity factor. When performing probabilistic analysis
of bearing capacity of strip footings, spatial variability must be
properly considered to avoid unconservative designs.
ACKNOWLEDGEMENT
The authors wish to acknowledge the support of (i) The
Australian Research Council Centre of Excellence for
Geotechnical Science and Engineering (ii) NSF Grant CMMI-
0970122 on "GOALI: Probabilistic Geomechanical Analysis in
the Exploitation of Unconventional Resources."
REFERENCES
Fenton, G. A., and Griffiths, D. V., 2008. Risk Assessment in
Geotechnical Engineering. John Wiley & Sons, New York.
Fenton, G. A., and Vanmarcke, E. H., (1990). Simulation of
random fields via local average subdivision. J Eng Mech,
ASCE, 116(8):1733
–
1749
Griffiths, D.V., and Fenton, G.A., Bearing capacity of spatially
random soil: The undrained clay Prandtl problem revisited,
Géotechnique, 54(4), 351--359, 2001.
Krabbenhøft, K., Lyamin, A.V., Hjiaj, M., and Sloan, S.W.
2005. A new discontinuous upper bound limit analysis
formulation. International Journal for Numerical Methods in
Engineering, 63: 1069-1088.
Lyamin, A.V., and Sloan, S.W. 2002a. Lower bound limit
analysis using non-linear programming. International
Journal for Numerical Methods in Engineering, 55: 573-
611.
Lyamin AV, Sloan SW. Upper bound limit analysis using linear
finite elements and non-linear programming. International
Journal for Numerical and Analytical Methods in
Geomechanics 2002b; 26:181
–
216.
Sloan, SW (1988) “Lower bound limit analysis using
finite
elements and linear programming”, Int J Numer Anal Meths
Geomech, 12(1), pp.61-77
Sloan, SW (1989) Upper bound limit analysis using finite
elements and linear programming. International Journal of
Numerical and Analytical Methods in Geomechanics, 13(3),
263-282.
Sloan SW, Kleeman PW. Upper bound limit analysis using
discontinuous velocity fields. Computer Methods in
Applied Mechanics and Engineering 1995; 127:293
–
314.
Smith IM, Griffiths DV. Programming the finite element
method. 4th edition, John Wiley & Sons: Chichester, 2004.


