468
Proceedings of the 18
th
International Conference on Soil Mechanics and Geotechnical Engineering, Paris 2013
unfrozen water content function; this function was calibrated
with the data for Fairbanks silt, Figure 5. The curve fitting
function is represented in Figure 5 by the continuous curve
(red). The unfrozen water curve is quite steep, and it is assumed
that the functions for freezing and thawing process are the same.
Table 1. Parameters and initial values in simulation
λ
κ
Initial
p
0
M
α
1
α
2
0.35
0.1
60 (kPa)
1.0
0.2
0.2
e
0
w*
0
w w
a
μ
β
0.85
0.08
0.325
6.0
0.3
0
-2.0
-1.5
-1.0
-0.5
0.0
0.5
0.05
0.10
0.15
0.20
0.25
0.30
0.35
UnfrozenWaterContent
Temperature(
o
C)
Figure 5 Unfrozen water content curve (experimental points from
Huang
et al
., 2004))
The soil column is 1 m tall and 0.05 m in width. The walls of
the column are adiabatic and rigid. The initial uniform
temperature is 1
C, and the initial vertical and horizontal
compressive stresses are 20 kPa and 10 kPa, respectively.
Initial and boundary conditions in terms of load and
temperature at the top of the column are shown in Figure 6. The
bottom of the column is fixed and the temperature is maintained
at 1
C throughout the process.
Figure 6 Boundary condition on top of the soil column
Figure 7 Temperature distribution at t=0 and t=1.3e7s
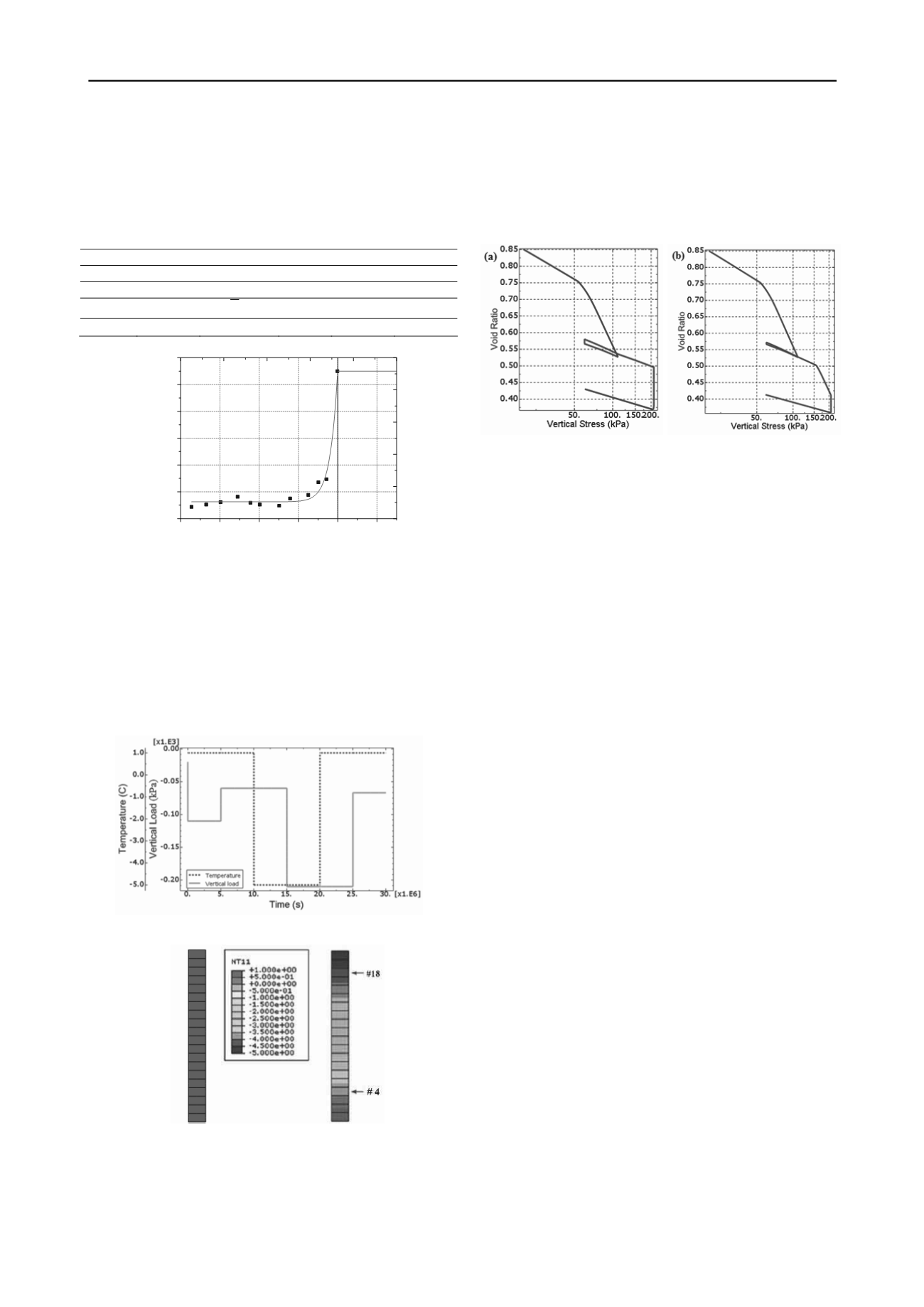
The relationship between void ratio and the vertical stress is
shown in Figure 8 for element #18 and #4. For element #18, the
temperature is about -4.5
C when the column reaches steady
state after freezing, having more pore ice and being stronger
than element #4 whose steady state temperature is around
-0.3
C. There is a substantial difference in the behavior of the
two elements during the loading segment from 60 to 210 kPa:
while element #18 behaves elastically, element #4 is elastic only
until the load reaches 160 kPa, and becomes elasto-plastic
afterward. Both elements experience additional settlement due
to thawing.
Figure 8 Compression for element (a) #18 and (b) #4
The model using the pore ice ratio as model parameter
captures freezing and thawing process for non-segregation soils
well. The parameter is related to the unfrozen water content
curve and is easy to obtain from tests. This constitutive model is
convenient to use, and it has been implemented in the FE
system. The model will be calibrated based on the test data
available, and it will be applied to solve practical boundary
value problems.
The model can be used as tool to predict the behavior of
soil subjected to freezing and thawing as long as no ice lenses
ce, construction
using artificial ground freezing. The model will be extended to
inc
investigation of soil heave by a large diameter chilled gas pipeline
a.
Journal of Cold Regions
. 2006. Frost heave modeling using
porosity rate function.
International Journal for Numerical and
in Geomechanics
, 30 (8), 703-722.
W. 2010. Experimental study of a pseudo-
Shas
a
are formed. Such problems include, for instan
lude ice lens formation in frost-susceptible soils.
5 ACKNOWLEDGEMENTS
The work presented in this paper was supported by the Army
Research Office, grant No. W911NF-08-1-0376. This support
is greatly appreciated.
6 REFERENCES
Alonso E.E., Gens A. and Josa A. 1990. A constitutive model for
partially saturated soils.
Géotechnique
, 40 (3), 405-430.
Huang S.L., Bray M.T., Akagawa S., and Fukuda M. 2004. Field
experiment, Fairbanks, Alask
Engineering
, 18 (1), 2-34.
Lee M.Y., Fossum A., Costiin L.S. and Bronowski D. 2002. Frozen soil
material testing and constitutive modeling.
Sandia National
Laboratories Report
2002-0524.
Michalowski R.L. and Zhu M
Analytical Methods
Qi J., Hu W. and Ma
preconsolidation pressure in frozen soils.
Cold Regions Science
and Technology
, 60, 230-233.
Roscoe K.H. and Burland J.B. 1968. On the generalized stress strain
behavior of wet clay. In
Engineering Plasticity,
eds. J. Heyman and
F.A. Leckie; Cambridge University Press, 535-609.
tri A. and Sanchez M. 2012. Mechanical modeling of frozen soils
incorporating the effect of cryogenic suction and temperature.
GeoCongress 2012: State of the Art and Practice in Geotechnical
Engineering
, 2492-2501.
Wei L., Hong F. and He W. 2011. Research on visco-elastic-plastic
creep model of artificially frozen soil under high confining
pressures.
Cold Regions Science and Technology
, 65 (2), 219-225.


