2247
Technical Committee 208 /
Comité technique 208
0.25B
CX
B, 1.05H
CY
3H, 0.80
N
d
1.25. In the
widely used limit equilibrium methods of slope analysis, the
potential slip surface and the sliding mass are divided into
segments or slices. The FOS, (F) is related to the total height of
the slope H, the effective subsoil parameters c
/
,
/
and
, the
pore pressure ratio r
u
(= u/
h), the individual slices of width b
i
,
height h
i
and
-the inclination of slice on the failure arc with
the horizontal, by the following equation (Bishop, 1955)
:
n
1i
i
i
i
n
1i
/
i
i
/
u
i
i
i
/
sinα
H
h
H
b
F
tanφ
tanα 1
secα
tanφ r 1
H
h
H
b
H
b
γH
c
F
A modest population size (n) of 20 is adopted. The design
variables of the fittest population genre (local FOS
min.
) is
perturbed sequentially by a factor
k in both directions,
resulting in 2(2
3
-1) =14 ‘offsprings’.
Initial value of
k is fixed
at 5% of the search interval
for each variable after some initial
trials. Hence,
k works out to be 3.75 [=.05x{(60.00)-(-15.00)}]
for x
i
-the abscissa of the slip-circle centre, 3.225 {=.05x(94.50-
30.00)} for y
i
-the ordinate of the slip-circle centre and 0.0225
{=.05x(1.25-0.80)} for z
i
-the depth factor of the slide (refer
Fig.2).
This
k is further shrinked in successive generations
by multiplying it with the size reduction parameter,
i
=G
i
(1-
r)
(Gi-1)
.
Again, the objective function evaluations are made for
the perturbed individuals and the minimum of these 14 points is
located. This local minimum point (offspring) acts as the
‘mother’ of the next generation, and the corresponding design
variables acts as the mean of the search space of next
generation.
0.000001
0.00001
0.0001
0.001
0.01
0.1
1
10
100
1000
0
2
4
6
8
10
12
14
16
18
20
22
24
26
28
30
32
34
36
38
40
42
44
46
48
50
k
Generation Number
Delta k (CY)
Delta k (Nd)
Search space exploitation parameter
-0.5
0.0
0.5
1.0
1.5
2.0
2.5
3.0
3.5
4.0
0
4
8
12
16
20
24
28
32
36
40
44
48
k
GenerationNumber
Delta k (CX)
-0.01
-0.006
-0.002
4
8
12
16
20
24
28
32
36
40
44
48
k
GenerationNumber
Fig.2. Search space exploitation in line with Design of Experiments.
0.0
0.2
0.4
0.6
0.8
1.0
0
2
4
6
8
10
12
14
16
18
20
22
24
26
28
30
32
34
36
38
40
42
44
46
48
50
i
=G
i
(1-r)
(G
i
-1)
Generation Number
Bounds of Search Space =x
f(i-1)min.
(+/- )
i
x
x
f(i-1)min.
Limit from mean of
search space
decaying with
sucessive generations
Geometric Decay
Constant : r=0.1
Exploration of search space
Fig.3. Search space exploration analogous to simulated annealing
schedule by contraction of search boundary in successive generations.
-15
-5
5
15
25
35
45
55
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
Design Variable CX
Random Population Species (n)
CX (1st Generation)
Perturbed CX (1st Generation)
CX (2nd Generation)
CX (50th Generation)
Perturbed CX (50th Generation)
Fig.4. Initial deterministic search space turns heuristic at 2
nd
Generation-
a quick shift towards the best part of search space.
-20
-10
0
10
20
30
40
50
60
0
2
4
6
8
10
12
14
16
18
20
22
24
26
28
30
32
34
36
38
40
42
44
46
48
50
Design Variable,CX
Generation No.
Variable CX-CENTRAL
Dynamic Upper Boundary of CX
Dynamic Lower Boundary of CX
a
-0.55
-0.45
-0.35
-0.25
-0.15
4
6
8
10
12
14
16
18
20
22
24
26
28
30
32
34
36
38
40
42
44
46
48
50
DesignVariable, CX
Generation Number
0
40
80
120
160
200
1
3
5
7
9
11
13
15
17
19
21
23
25
27
29
31
33
35
37
39
41
43
45
47
49
Design Variable,
CY
Generation Number
CY-CENTRAL
Dynamic Upper Boundary of CY
Dynamic Lower Boundary of CY
b
0.0
0.4
0.8
1.2
1.6
2.0
1
3
5
7
9
11
13
15
17
19
21
23
25
27
29
31
33
35
37
39
41
43
45
47
49
Design Variable Nd
Generation Number
Nd-CENTRAL
Dynamic Upper Boundary of Nd
Dynamic Lower Boundary of Nd
c
60
70
80
90
100
0
2
4
6
8
10
12
14
16
18
20
22
24
26
28
30
32
34
36
38
40
42
44
46
48
50
CY & Raius of
Critical Circle
(R)
Generation Number
CY Radius of Critical Circle (R)
d
Fig.5. Robust movement of variable bounds along with the central value
of tri-variables (CX, CY, N
d
) shown in (a), (b), (c) respectively. In (a), a
further close-up view from 2
nd
generation is shown.
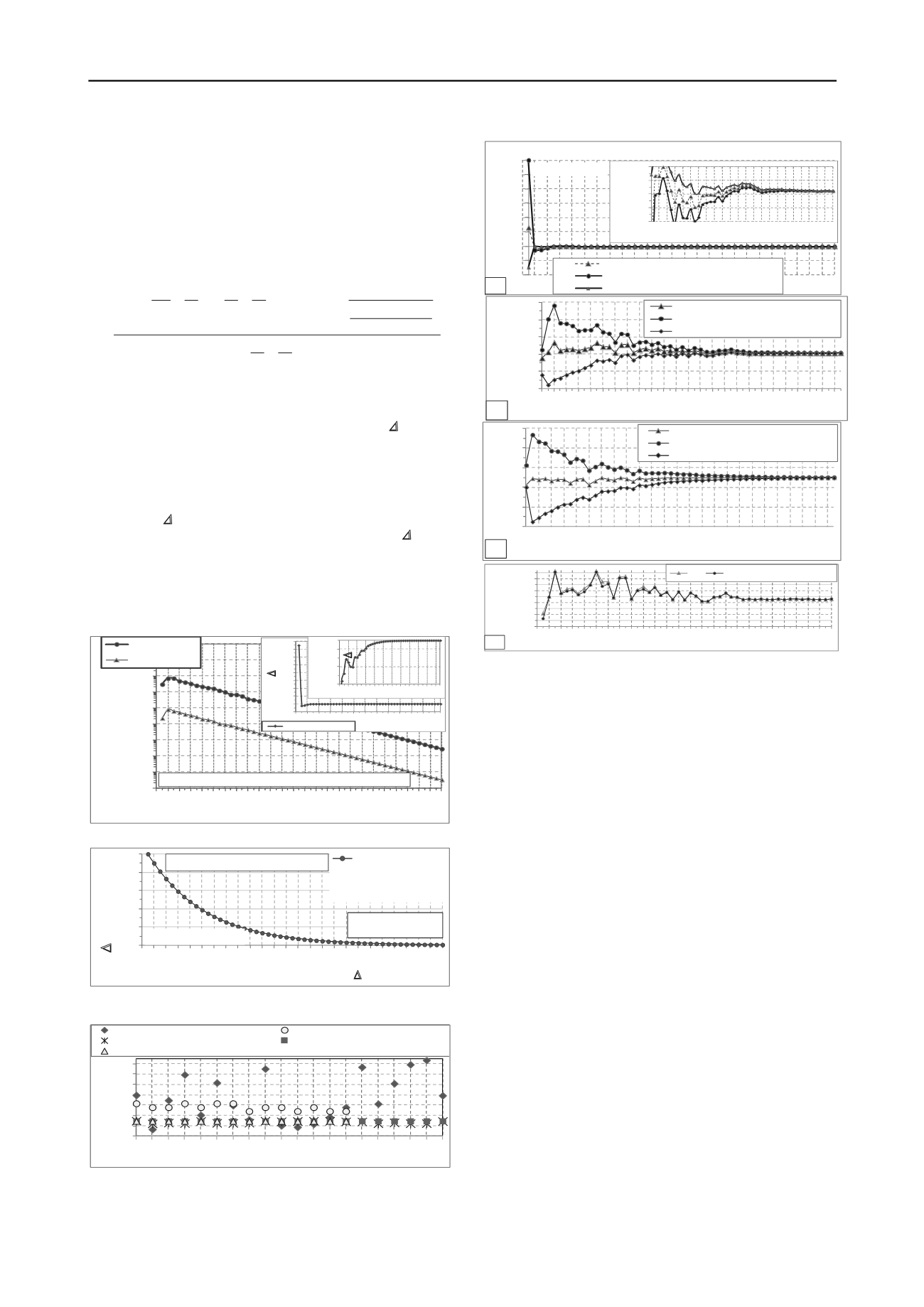
From (d) it emerges
that R
CY for critical circle.
4 COMPUTER SIMULATION & GRAPHICAL
DEPICTION OF THE SMART ADAPTIVE PROCESS
Fig.3 shows how the limit of search boundary shrinks towards
the mean (that is, the central value or the best point of the
preceding generation) of the search space. Fig.4 shows a typical
result of 1
st
, 2
nd
and 50
th
randomly generated population along-
with the perturbed population set of 1
st
and 50
th
generations.
It
may be noted that initial wide deterministic search space turns
heuristic at 2
nd
generation with a quick shift towards the best
part of search space.
Fig.5(a) to (c) depict the robust movement
of variable bounds along with the central value of the three
design variables (CX, CY & N
d
), illustrating the generate-and-
test heuristic search technique that exploit domain-specific
knowledge. It emerged that whatever be the initial search
bounds specified deterministically, the algorithm adjusts itself
to move to the best part of search space in the immediate 2
nd
generation. It is revealed that the
bounds of CX are drastically
reduced (Fig.5a), and that of CY & N
d
are radically expanded
(Fig.5b&c) in immediate 2
nd
generation
, and thereafter the
bounds move steadily with successive generations that are
guided by the mean of the search space, while maintaining a
heuristic character. It emerged that the value of radius of critical
circle (R) almost merges with the ordinate (CY) of the critical
slip circle (Fig.5d). Fig.6 depicts the change in average fitness,
standard deviation and variance of fitness function of
population with successive generations. The variance of fitness
decreases steadily with increasing generations maintaining its
randomness. Fig.7 shows the maximum fitness of each
generation vs. search space size reduction parameter in log-log
scale. Fig.8 portrays the stochastic movement of Min. FOS in
successive generations to converge to global optimum, wherein
results of five simulation runs are superimposed. The inherent


