1253
Technical Committee 202 /
Comité technique 202
(a)
(b)
(c)
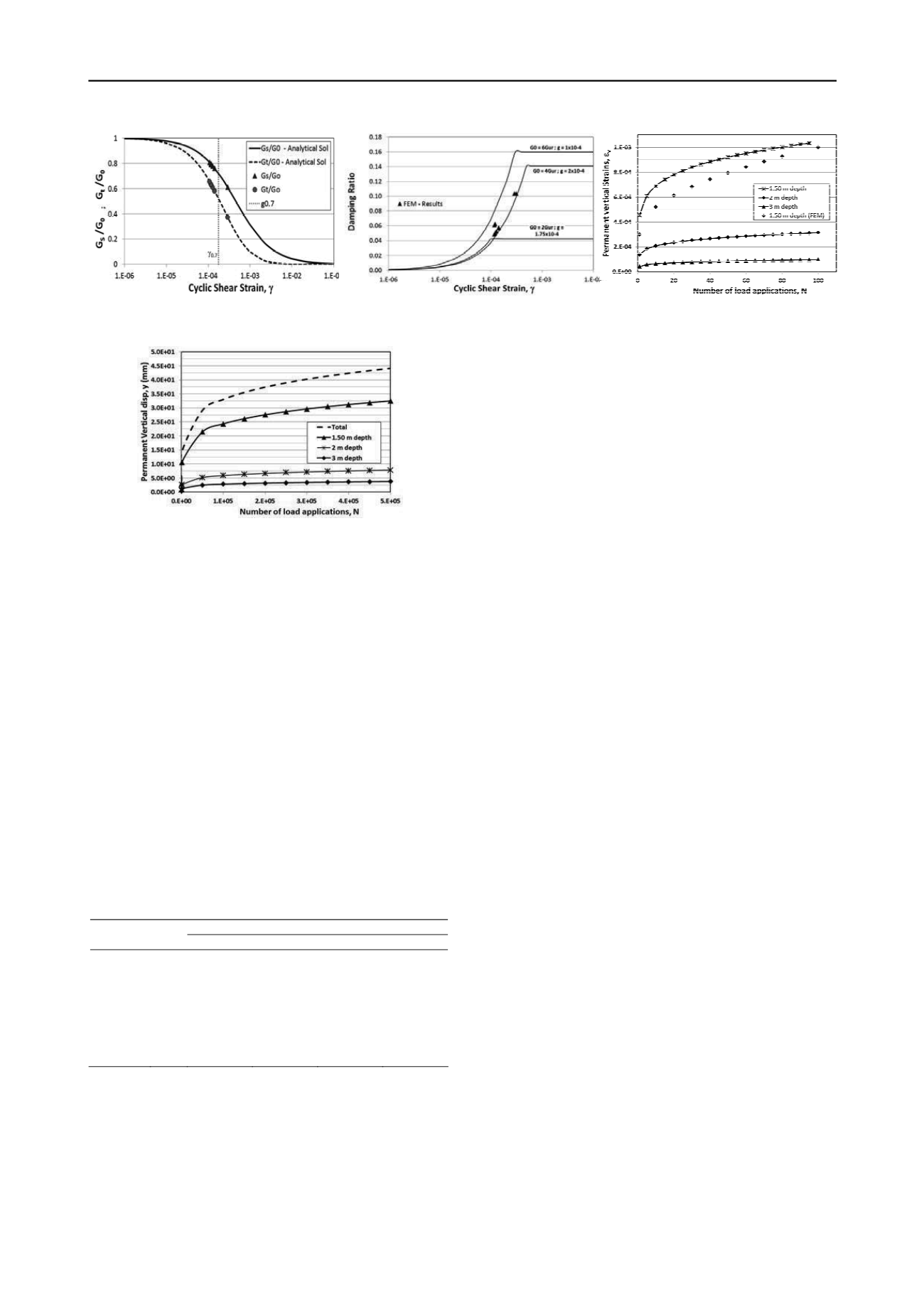
Figure 6. (a) Shear moduli variation with strain level; (b) damping ratio according to cyclic shear strain; (c) cumulative strain with load repetitions.
Figure 7. Cumulative vertical displacement (rutting depth).
q
f
= static failure deviator stress; N = number of repeated load
applications; and a, m, n and b are constant largely dependant
on soil properties, which could be assumed for the soft soil
analyzed here, according to those values suggested by Li and
Selig (1996): a = 1.2; b = 0.18; m = 2.4; n = 1.
Considering the results of q
is
, q
d
and q
f
depicted in Figures
4b and 5, the cumulative plastic strain is calculated at different
depths according to the Equation 6. In Figure 6c is observed that
results from finite element model fit reasonably well with the
empirical equation proposed by Chai and Miura (2000)
considering N = 100. Otherwise, Figure 7 shows a cumulative
permanent deflection of about 45 mm (rut depth), given from
Equation 6 for a number of repeated load applications up to N =
5·10
5
, and considering the thickness of soft soil influenced by
the cyclic load. Such results suppose an unallowable level of
rutting failure, even for a low trafficked road as its typical
thresholds range from 10 to 15 mm for N greater than 10
6
.
Possible solutions for pavements based over such soft soil might
be achieved by means of deep ground improvement, which
could overcome the detrimental effects of the induced deviator
stress and excess pore pressure throughout the depth of load
influence (Elias et al. 2004, Sonderman and Wehr 2004).
Table 3. Results of subgrade soil stiffness at 1.5 m depth.
Number of load repetitions
Parameter Unit
10
30
50
100
OCR
-
1.2
1.4
1.47
1.5
-
2.90E-04
1.16E-04
1.20E-04
1.07E-04
G
S
/G
0
-
0.61
0.80
0.79
0.81
G
t
/G
0
-
0.37
0.63
0.63
0.66
G
S
kPa
27473
35851
35601
29485
-
0.891
0.844
0.822
0.794
E
ur
*
kPa
63661
78635
73191
58551
E
50
*
kPa
25464
31454
29276
23421
*final stiffness
4 CONCLUSION
The theoretical procedure presented by means of finite element
modelling has shown that deep soft soils might be decisive to
long term behavior of flexible pavements, especially in the
cases when shallow treatments of subgrade would be
uneconomic or inefficient. Deep soil treatments should be
applied to achieve an allowable capacity of soft soils up to
minimum depth of about 6 m, otherwise maintenance cost of
pavements might be excessive. The analysis presented has
included the response of soft subgrade layers under static and
cyclic loading taking into account the influence of small-strain
levels on the soil stiffness; the results fit reasonably well with
the analytical solution of hysteretic damping ratio presented by
Brinkgreve et al. (2007).
5 REFERENCES
Barksdale R. G. 1971. Compressive stress pulse times in flexible
pavements for use in dynamic testing. Highway Research Record
345. pp 32-44. Highway Research Board.
Benz T. 2006. Small-strain stiffness of soils and its numerical
consequences. Ph.d. thesis. Universität Stuttgart.
Brikgreve R. B. J. Kappert M.H. and Bonnier P.G. 2007. Hysteretic
damping in a small-strain stiffness model. NUMOG X. 737-742.
Chai J. C. and Miura N. 2000. Traffic load induced permanent
deformation of low road embankment on soft subsoil. Proceedings
of International Conference on Geotechnical and Geological
Engineering. CD Rom, Paper No. DE0239
Dobry R. and Vucetic M. 1987. Dynamic properties and seismic
response of soft clay deposits. Proc. International Symposium on
Geotechnical Engineering of soft soils. Mexico city. pp 51-87.
Duncan J.M. and Chang C.Y. 1970. Nonlinear analysis of stress and
strain in soil. J. Soil Mech. Found. Div. ASCE 96. 1629-1653.
Elias V. Welsh J. Warren J. Lukas R. Collin J.G. and Berg R.R. 2004.
Ground Improvement Methods. Participant Notebook. NHI Course
132034. FHWA NHI-04-001. Washington. D.C. 1022 pp.
Giroud J.P. and Noiray L. 1981. Geotextiles-reinforced unpaved road
design. ASCE. Journal of Geotech. Engg. 107(9). 1233-1253
Hardin B. O. and Drnevich V. P. 1972. Shear modulus and damping in
soils: Design equations and curves. Proc. ASCE: Journal of the Soil
Mechanics and Foundations Division. 98(SM7). 667-692.
Idriss I. M. Dobry R. and Singh R.D. 1978. Nonlinear behavior of soft
clays during cyclic loading. Journal of Geotech. Engg. ASCE. vol.
104. No. GT12. Dec. pp. 1427-1447.
Ishihara K. 1996. Soil Behaviour in Earthquake Geotechnics. Oxford
Engineering Science Series. Oxford University Press.
Korkiala-Tanttu L. and Laaksonen R. 2004. Modelling of the stress state
and deformations of APT tests. In Proc. of the 2nd Int. Conf. on
Accelerated Pavement Testing. Minnesota. Worel, B. 22 p.
Ladd C. C. 1991. Stability evaluation during stage construction. Journal
of Geotechnical Engineering. ASCE. Vol. 117. No. 4. pp. 541-615.
Li D. and Selig E.T. 1996. Cumulative plastic deformation for fine-
grained subgrade soils. Journal of Geotechnical Engineering.
ASCE. Vol. 122. No. 12. pp. 1006-1013.
Santos J. A. and Correia A.G. 2001. Reference threshold shear strain of
soil. Its application to obtain a unique strain-dependent shear
modulus curve for soil. Proc. 15
th
Int. Conf. on Soil Mechanics and
Geotechnical Engg. Istanbul. Vol 1. 267-270.
Schanz T. 1998. Zur Modellierung des Mechanischen Verhaltens von
Reibungsmaterialen. Habilitation. Stuttgart Universität.
Schanz T. Vermeer P.A. and Bonnier P.G. 1999. The hardening-soil
model: Formulation and verification. In R.B.J Brinkgreve. Beyond
2000 in Computational Geotechnics. Balkema. Rotterdam. 281-290.
Sonderman W. and Wehr W. 2004. Deep vibro techniques. In Moseley
M.P. and Kirsch K. eds. Ground Improvement 2
nd
edition. Spon
Press. London and New York.
Vucetic M. and Dobry R. 1991. Effect of soil plasticity on cyclic
response. Journal of Geotech. Engg. ASCE. vol. 117. pp. 89-107.


