1826
Proceedings of the 18
th
International Conference on Soil Mechanics and Geotechnical Engineering, Paris 2013
4 EXAMPLE OF APPLICATION
In the following the Eurocode EN 1997 will be applied in an
example and compared to the alternative method presented in
the previous section. The intention is to outline some issues the
authors consider as problematic in a simplistic way. The
example is thus very simplified and does not as such represent a
true case study.
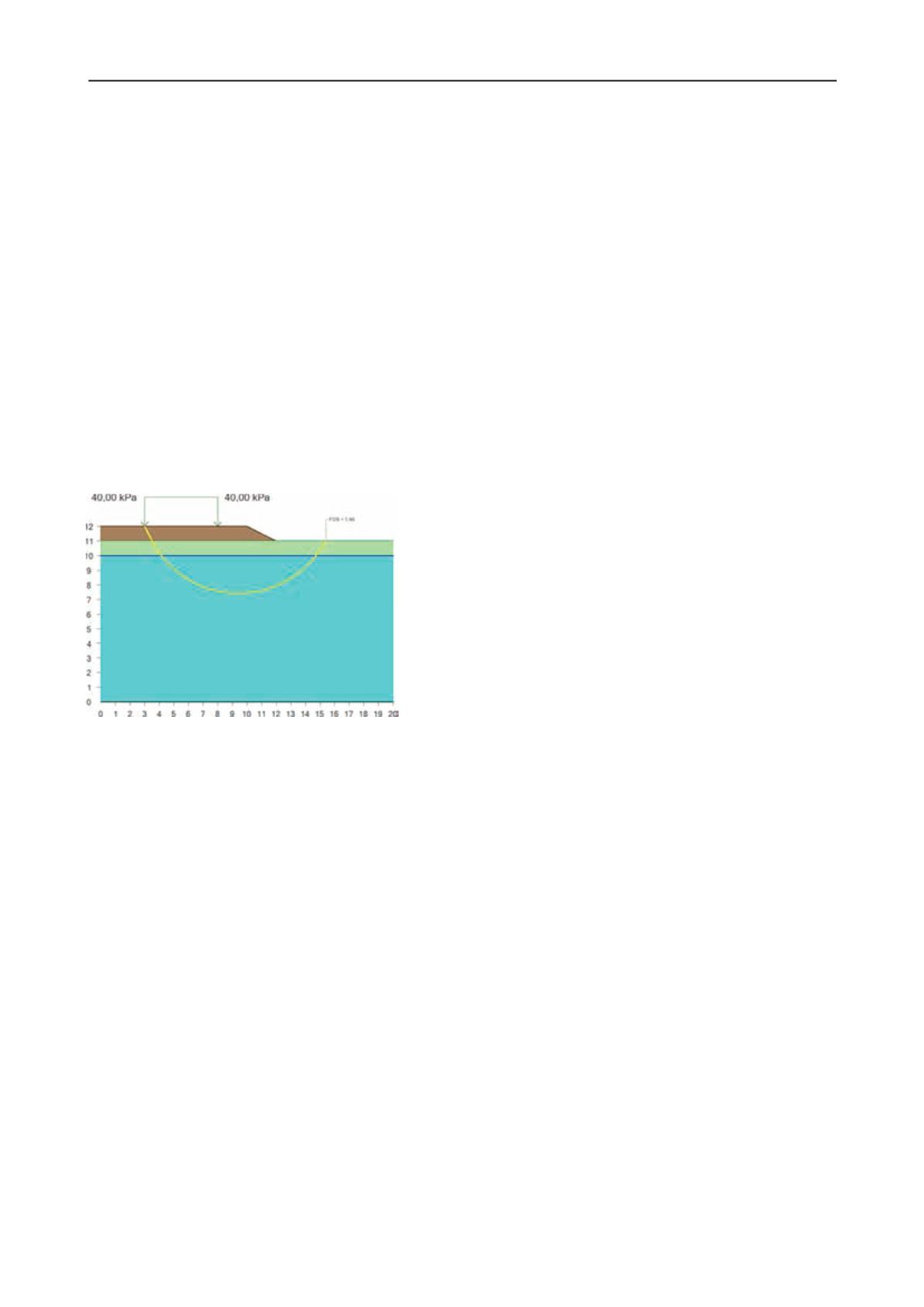
Let us consider the situation given in Figure 4. The soil
conditions are the following. A 1m thick embankment is laid
upon a dry crust layer. The unit weight of the embankment
material is
γ
= 20kN/m
3
and the characteristic friction angle is
φ
= 38
o
. The dry crust layer is 1m thick and has a unit weight of
γ
= 17kN/m
3
and the characteristic undrained shear strength is
30kPa. Under the dry crust there is a layer of soft clay with a
unit weight of
γ
= 16kN/m
3
and a characteristic undrained shear
strength of 10kPa at top of layer increasing with 1.4kPa/m
deapthwise. A 5m wide load of 40kPa is placed two meters
from the crest of the embankment. The problem in question is
much load driven. The total safety factor without any load is
around 4 while the 40kPa load decreases it to 1.46 for a circular
failure surface analysed by the Bishop method.
Figure 4. Geometry of the problem and calculated total factor of safety.
According to the recommended values to EN 1997 the partial
safety coefficient for effective stress strength a parameters is
γ
φ
’
=
γ
c’
= 1.25 and for total stress analysis
γ
cu
= 1.4. Applying these
yields for design values a friction angle of
φ
= 32
o
and
undrained strength values of 21.4kPa for the dry crust and
7.1kPa + 1kPa/m for the soft clay. If the load comes from a
permanent load, the recommended partial factor is 1.0 while it is
1.3 for variable loads. So in case of variable loads the design
value entering the calculation is 52kPa.
In case of a permanent load the resulting over dimensioning
factor is ODF = 1.04 indicating that the situation is safe. For the
variable load case the ODF reduces to ODF = 0.88. To have
enough safety, the characteristic initial value of undrained shear
strength of the clay should increase by some 30 % to 13kPa.
This corresponds to a total safety factor of 1.69 for the situation.
Is it then reasonable to require a higher safety for the variable
load case? A general argument in favour of this is that there
might be more uncertainty for the variable load than for the
permanent one. This is however not necessarily true. A typical
high variable load representative to embankment stability would
be train load from a heavy freight train. However, railway tracks
are classified and there is an upper load allowed for a certain
track part. So the characteristic load is rather a maximum load.
In such cases the partial factor for action should rather be
calculated using a log-normal distribution than a normal
distribution. Also, there will always be uncertainty also in the
permanent load which is disregarded in EN 1997-1. It is perhaps
also more important to consider the consequences of failure. A
permanent load might come from a residential building. The
consequence of failure might thus be very severe with lots of
casualties. On the other hand if the variable load is due to a
freight train carrying e.g. iron ore the consequences of failure in
an uninhabited area are perhaps not that severe – at least the risk
for loss of lives is minor. However, if a train with toxic material
goes through an inhabitant area the consequences of failure are
of course harsh.
The alternative approach presented in 3.4 allows for such
considerations. It is emphasized that the results presented are
aimed to give an example how safety could be applied. The
assumed distributions, variations and target reliabilities needs of
course careful consideration. However, if one assumes a
variability of 0.1 for friction and 0.2 for the undrained shear
strength one finds, that the recommended partial safety factors
γ
φ
’
=
γ
c’
= 1.25 and
γ
cu
= 1.4 in EN 1997-1 corresponds to the
calculated one at approximately a load ratio of 80% assuming
no additional uncertainty. While this is a very high load ratio its
use is justified by the sake of comparison and the fact that the
case is highly load driven. For the alternative approach
corresponding safety factors for same
β
would be
γ
φ
’
=
γ
c’
= 1.52
and
γ
cu
= 1.73. Applying this as a load factor on unity yields an
ODF = 0.84. Now to have enough safety the undrained shear
strength of the clay would need to increase to 13.3 kPa. This
corresponds to on total safety factor of 1.71 i.e. close to the
partial safety factor used for the undrained shear strength.
Similarly the total safety requirement for a high consequence
class (RC3) would be close to 2.0 and for a minor consequence
class (RC1) approximately 1.5.
5 CONCLUSIONS
The partial safety factor approach in EN 1997-1 adopted in most
European countries for slope stability is reviewed. The author’s
conclusions are that risk and consequence of failure are not
necessarily properly accounted for. For situation with no
variable loads the safety level applied in EN 1997-1 does not
correspond to the implied reliability index, but is below that.
Also the consequence of failure is not properly addressed, as the
load factor in EN 1990 have a negliable affect to safety for
some slope stability problems. An alternative approach is
presented, where all uncertainty is placed on the material partial
safety factor and the consequence of failure is accounted by
calculating the material safety factors separately for different
consequence classes with different target reliability index
values.
6 REFERENCES
Abramson, L., Lee, T. S., Sharma, S. and Boyce, G. M. 2002. Slope
stability and stabilization methods. John Wiley & Sons, Inc.
European committee for standardization, CEN. 2002. EN 1990
Eurocode: Basis of structural design. Brussels: European committee
for standardization.
European committee for standardization, CEN. 2004. EN 1997-1
Eurocode 7: Geotechnical design. Part 1:General rules. Brussels:
European committee for standardization.
Frank, R., Bauduin, C., Driscoll, M., Kavvadas, M., Krebs, Ovesen, N.,
Orr, T., Scuppener, B. 2004. Designer’s guide to EN 1997-1
Eurocode 7: Geotechnical design-General rules. London: Thomas
Telford Ltd.
Leroueil, S. Magnan, J.-P. and Tavenas, F. 1990. Embankments of soft
clays. Ellis Horwood.Darcy H. 1856.
Les fontaines publiques de la
ville de Dijon
. Dalmont, Paris.
Poutanen T., 2011. Calculation of partial safety factors, Applications of
Statistics and Probability in Civil Engineering – Faber, Köhler &
Nishijima (eds), Taylor & Francis Group, London


