1557
Technical Committee 203 /
Comité technique 203
The horizontal forces are the hydrostatic loading
U
h
, the active
earth pressure acting on the upstream vertical face of the sliding
block
E
A
and the passive earth pressure acting on the
downstream face
E
P
. They were computed to be:
U
h
100
MN m
E
P
E
A
15
MN m
(11)
The factor of safety against block sliding is therefore
FS
S
u
U
h
E
A
E
P
144
MN m
100
MN m
15
MN m
1.6
(12)
Therefore, the dam was stable without the buttress. To stress the
importance of this conclusions - based on crude estimations and
simple analyses - a back-calculation can be performed. Failure
might occurr if the shear strength in the sliding plane were
85
MN/m
. After eqn. (10), this would imply a
s
u
/
p
ratio 0.108,
which inserted in eqn. (6) would in turn mean that the dam is
founded on a continuous, horizontal layer with (N
1
)
60
= 10.
4 NUMERICAL ANALYSIS
4.1
Hypotheses
A simple 2D pushover analysis of the dam was performed
numerically using Plaxis. The model geometry and mesh
reproduce Figure 1; material parameters were estimated based
on published information only (e.g., Barchiesi et al 2006,
Carmona et al 2004) and are described below.
A continuous horizontal layer 4m thick was assumed to be
located 30m below the foundation level of the dam, running
throughout the model. Construction stages were not simulated;
the dam was activated in one drained loading step. For the
pushover analy-ses, all materials were assumed drained except
for the sand layer, where undrained behavior was enforced
numerically. Due to its permeability and thickness, it was
assumed that any cohesive layer would complete primary
consolidation during the construction of the dam.
4.2
Description of the constitutive model
The model focused on the progressive sliding deformations of
the weak layer; only this material was modelled accurately
using a constitutive model developed by Sfriso for the
monotonic shear of sands (Sfriso and Weber 2010).
The model is designed to simulate the behavior of sands in the
whole stress and strain range of engineering interest, using state
independent material parameters. The formulation is based on
effective stresses, pressure dependent hyperelasticity, non-
associative elasto-plasticity, an isotropic hardening law and
Rowe’s stress-dilatancy theory (Sfriso and Weber 2010).
4.3
Material parameters and loading
Shear modulus was computed using the Hardin and Drnevich
(1972) expression
G
c
s
c
e
e
2
1
e
p
100
kPa
m
100
kPa
(13)
where
c
s
= 840,
c
e
= 2.17,
m
= 0.50 and
p
is mean pressure.
A Matsuoka-Nakai yield function was employed, calibrated for
triaxial compression with the friction angle obtained using eqn.
(4), where
Δ
ϕ
= 3º,
ϕ
c
= 31º,
p
r
= 55, and
R
= 2º.
A major issue is the estimation of relative density. A back-
calculation of eqn. (5) to yield a
s
u
/
p
= 0.18, as used in the rigid
body calculations, would imply
D
r
= 46%, a somewhat high
value to expect undrained yielding (Sfriso 2010). Therefore,
numerical analyses were run adoptng
D
r
in the range 30% -
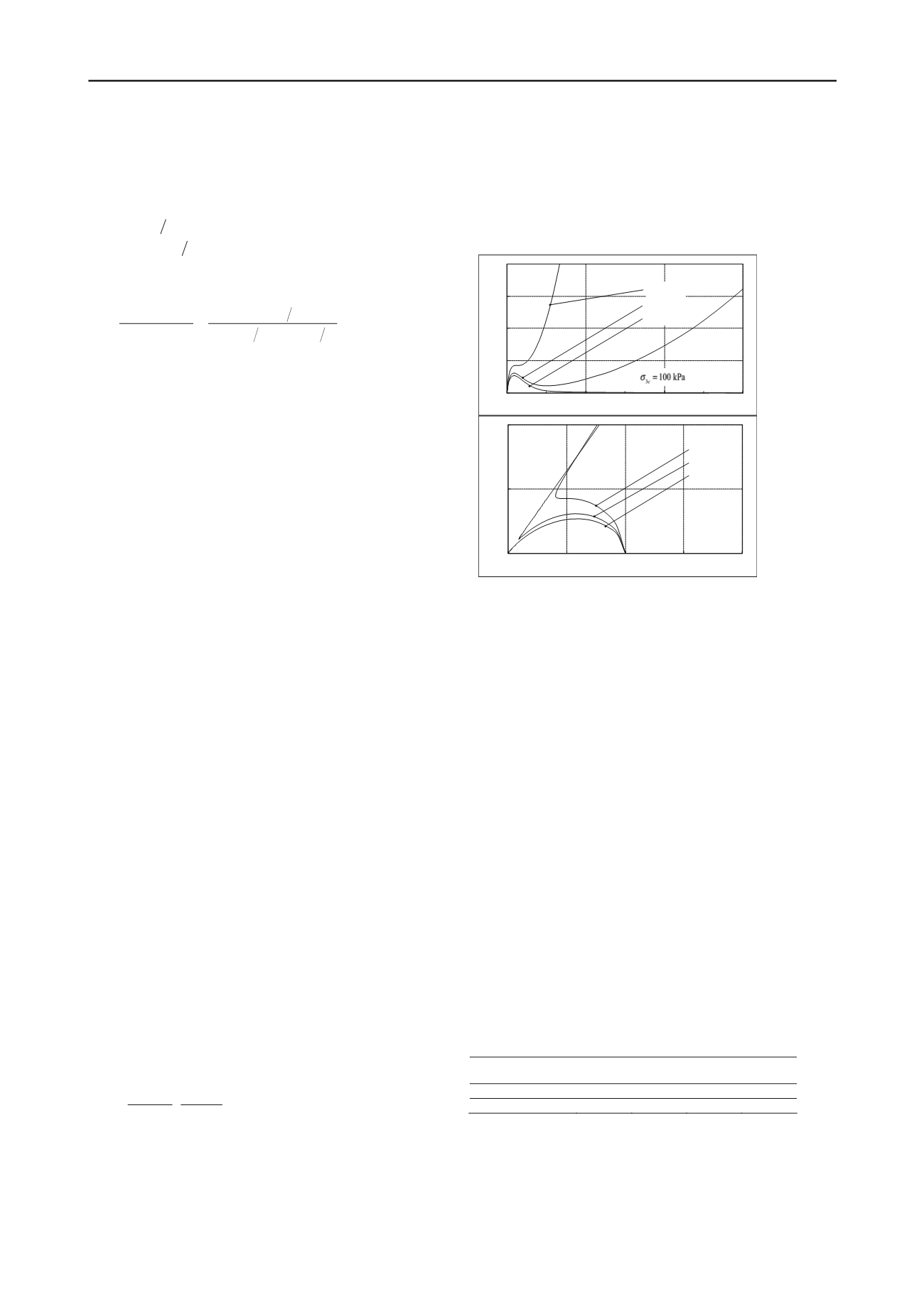
45%. The resulting stress-strain and p-q plots for undrained
triaxial compression are shown in Figure 2 for the
D
r
= 15%,
25% and 50% and for a confining pressure of 100kPa.
0
50
100
150
200
0.00
0.01
0.02
0.03
1
50%
25%
15%
r
r
r
D
D
D
kPa
q
0
50
100
0
50
100
150
200
50%
25%
15%
r
r
r
D
D
D
kPa
p
kPa
q
Figure 2. p-q plot of the simulated undrained compression of the weak
layers underlying the foundation of Potrerillos dam.
The HSM model available in Plaxis (Brinkreve et al 2006) was
employed for the remaining foundation and dam. Only the
elastic parameters of the alluvium were of real interest for the
analysis of progressive failure. However, due to the formulation
of the HSM model, strength parameters do impact on the elastic
response, and therefore all parameters required realistic values
to yield a reliable prediction. The adopted values were
E
50
ref
= 80MPa,
E
oed
ref
= 90MPa,
E
ur
ref
= 200MPa, ν = 0.20,
c
= 1 kPa,
ϕ
= 45º, ψ
= 10º
,
OCR = 1.0. For the definitions of
these parameters see Brinkgreve et al (2006).
4.4
Factor of safety
The numerical computation of factor of safety is routinely
performed in Plaxis using the "phi-c reduction" concept
(Brinkreve et al 2006). For user defined soil models, Plaxis does
not support the use of the "phi-c reduction" scheme and
therefore an alternative definition must be attempted.
In this contribution, two "factors of safety" are defined: i) FS
1
:
the minimum factor augmenting the horizontal water load
U
h
and yielding non-convergence of the field equations of the
boundary value problem; and ii) FS
2
: the factor augmenting
U
h
to yield a permanent displacement of 1m at the base of the
concrete wall. The vertical component
U
v
remained unchanged
during both analyses. The results are shown in Table 1.
Table 1. Factors of safety computed for various relative densities.
Relative density
30%
35%
40%
45%
FS
1
1.39
2.45
-
-
FS
2
1.16
1.35
1.64
1.88
The large differences that can be observed between the two
factors of safety is due to the fact that the constitutive model
goes beyond the phase transformation plateau into the dilating
phase, as shown in Figure 2. FS
1
is not informed for
D
r
= 40%
and 45% because the failure mode was sliding at the foundation
level of the dam instead of deep sliding along the sand layer.


