2223
Technical Committee 208 /
Comité technique 208
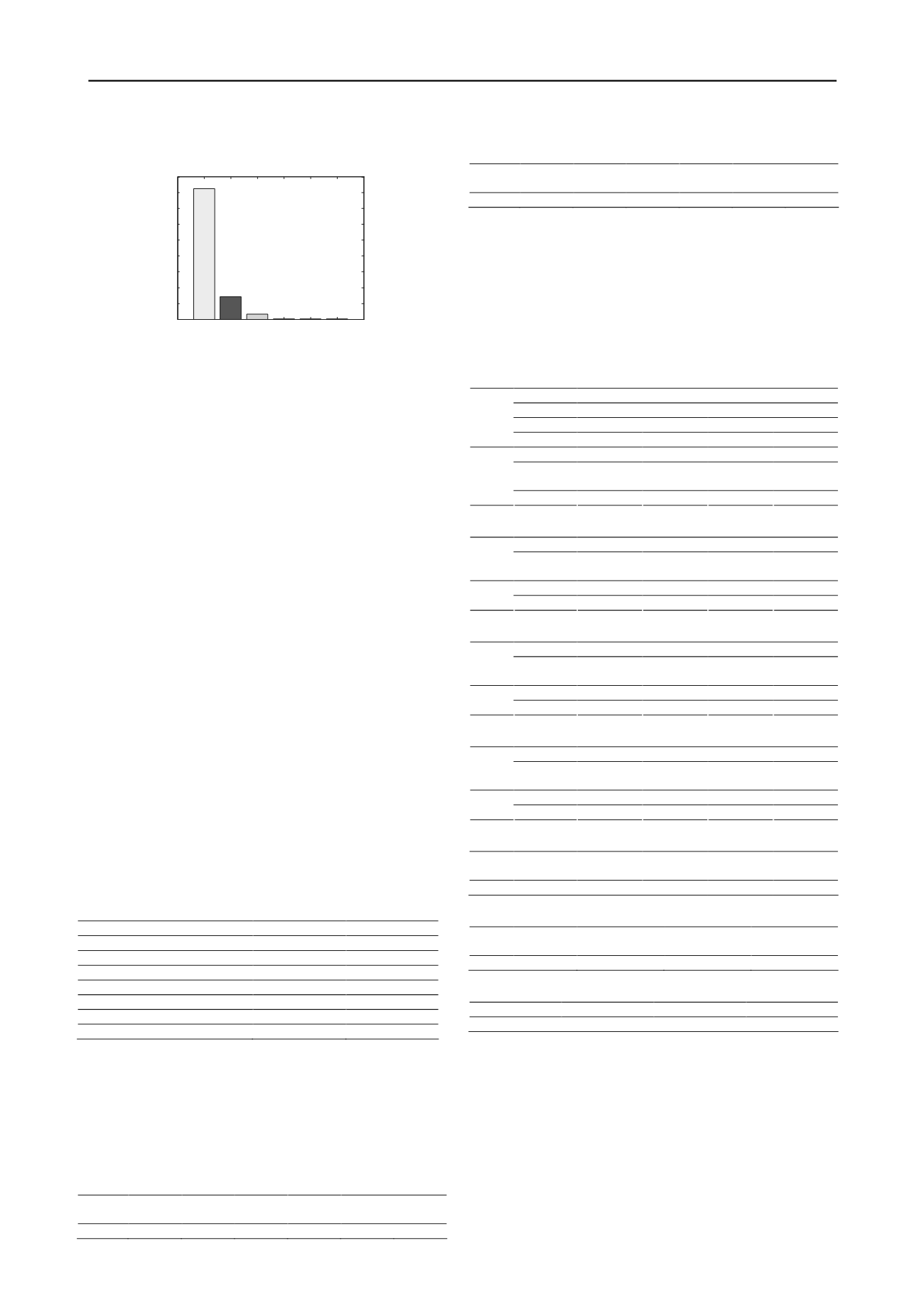
PGA are obtained based on inference of Bayesian network, as
shown in Fig. 5
0-8 8-16 16-24 24-32 32-40 40-48
0
0.1
0.2
0.3
0.4
0.5
0.6
0.7
0.8
0.9
PGA (10
-2
g)
Probability
Fig. 5 Specification of the discrete probabilities of PGA.
4.2
Landslide hazard
Approaches developed to assess the stability of slopes during
earthquake fall into three general categories: (1) pseudo-static
analysis, (2) stress-deformation analysis, and (3) permanent dis-
placement analysis. In this paper, dynamic performance of
slopes is modelled using permanent displacement analysis de-
veloped by Newmark (1965). The critical acceleration of a land-
slide block can be calculated by
a
c
= (FS-
1) g sinα
(8)
where
FS
is the static factor of safety;
g
is the acceleration of
Earth’s gravity; and
α
is the angle of the sliding surface, which
can generally the approximated as the slope angle.
The static factor of safety (
FS
) for an infinite slope is
FS =
c’/(γz sin
α
cosa)+(1-
m γ
w
/
γ)tanφ’
/tanα
(9)
where
c’
and
φ’
are the effective cohesion and friction angle of
the soil;
z
is the depth of the failure surface;
α
is the slope angle;
γ
is the soil unit weight; and
γ
w
is the specific weight of water.
In the present study, the Newmark displacement is estimated
using Eq. 10 reported by Ambraseys and Menu (1998):
Log D
n
= 0.9+log[(1-a
c
/a
max
)
2.53
(a
c
/a
max
)
-1.09
]
(10)
where
D
n
is the Newmark displacement in centimeters,
a
c
and
a
max
are critical acceleration and peak ground acceleration in
g’s
respectively.
The probability of slope failure as a function of Newmark
displacement, as described by Jibson
et al
(2000) is estimated
using the following equation
P(f) = 0.335×[1-exp (-0.048× D
n
1.565
)]
(11)
The soil and slope properties used in this study are shown in
Table 2.
Table 2. Soil and slope properties.
Variable
Mean
St. Dev
c’
(N/m
2
)
10 000
2 000
φ’
(degree)
30
2
z
(m)
2.5
0
α
(degree)
35
0
γ
(N/m
3
)
27 500
0
γ
w
(N/m
3
)
10 000
0
m
0.4
0
The probabilities of slope failure computed by Eq. 11 for
various ranges of PGA are listed in Table 3. Countermeasures
made to landslide could reduce risk. Specifically, active actions
can reduce the probability of slope failure, passive actions and
warning system can reduce the vulnerability of element(s) at
risk. The assumed probability of slope failure when active ac-
tions are taken is shown in Table 4.
Table 3. The probability of slope failure
PGA
(10
-2
g)
0-8
8-16 16-24 24-32 32-40 40-48
P(f)
0.124 0.256 0.305 0.328 0.339 0.346
Table 4. The probability of slope failure when active actions are taken
PGA
(10
-2
g)
0-8
8-16 16-24 24-32 32-40 40-48
P(f)
0.025 0.03 0.035 0.04 0.045 0.05
4.3
Other nodes
In the case of a building subjected to a multi-hazard situation
involving additive load effects (e.g. earthquake + landslide), the
damage will be increased. Herein, the conditional probabilities
of building damage are modified from Einstein
et al
(2010). For
other nodes, we adopt from Einstein
et al
(2010). These prob-
abilities (conditional probabilities) are shown in Tables 5-11.
Table 5. Four combinations of conditional probabilities of Building
damage
PGA
0-0.08
Measure
Yes
Decision
Passive
Active
Parent
nodes
Landslide Happens Does not
Happens Does not
No damage
0.4
0.1
0.52
0.1
Some
damage
0.3
0.1
0.43
0.1
Build-
ing
damage
Collapse
0.3
0.8
0.05
0.8
Table 6. Four combinations of conditional probabilities of Measure
Alarm
Yes
Parent
nodes Decision Passive
Active
No
action
Warning
system
Yes
0
0
0
1
Measure No
1
1
1
0
Table 7. Four combinations of conditional probabilities of Alarm
Landslide
Happens
Parent
nodes Decision Passive
Active
No
action
Warning
system
Yes
0.5
0.5
0.5
0.9
Alarm No
0.5
0.5
0.5
0.1
Table 8. Four combinations of conditional probabilities of Alarm
Landslide
Happens
Parent
nodes Decision Passive
Active
No
action
Warning
system
Yes
0.5
0.5
0.5
0.9
Alarm No
0.5
0.5
0.5
0.1
Table 9. Conditional probabilities of Cost
Parent
nodes Decision Passive
Active
No
action
Warning
system
Cost
-1250
-2000
0
-500
Table 10. Conditional probabilities of Utility
Parent
nodes Damage No damage Some damage
Collapse
Utilities
0
-10000
-20000
Table 11. Conditional probabilities of Cost_measure
Parent nodes
Measure
Yes
No
Cost_measure
-1000
0
5 RESULTS
The results obtained using the described Bayesian network of
the entire risk assessment and decision are shown in Fig. 6. Dif-
ferent mitigation measures result in different utilities. The warn-
ing system, showing the lowest (negative) utility is the optimal
mitigation measure.


